Full solution
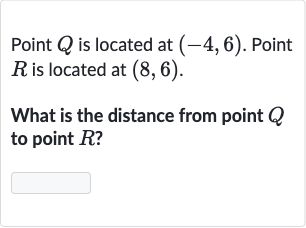
Q. Point is located at . Point is located at .What is the distance from point to point ?
- Identify Coordinates: Identify the coordinates of points and . Point is at and Point is at .
- Recognize Same Y-coordinate: Recognize that the points share the same -coordinate.Since both points have a -coordinate of , they lie on a horizontal line.
- Use Distance Formula: Use the distance formula for points on a horizontal line.The distance between two points on a horizontal line is the absolute difference between their x-coordinates.Distance =
- Substitute X-coordinates: Substitute the -coordinates of points and into the distance formula.Distance =
- Calculate Absolute Difference: Calculate the absolute difference.Distance = Distance =
- Simplify Absolute Value: Simplify the absolute value. Distance =
More problems from Find the length of the transverse or conjugate axes of a hyperbola
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help