Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

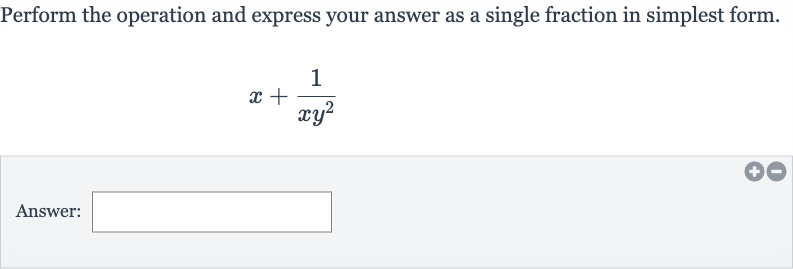
Perform the operation and express your answer as a single fraction in simplest form. x+(1)/(xy^(2)) Answer:

Perform the operation and express your answer as a single fraction in simplest form.\newlinex+1xy2 x+\frac{1}{x y^{2}} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Roots of rational numbersright chevron icon

Full solution

Q. Perform the operation and express your answer as a single fraction in simplest form.\newlinex+1xy2 x+\frac{1}{x y^{2}} \newlineAnswer:
  1. Identify Common Denominator: Identify the common denominator for the two terms.\newlineSince the first term xx has an implicit denominator of 11 and the second term has a denominator of xy2xy^2, the common denominator is xy2xy^2.
  2. Express First Term: Express the first term xx with the common denominator xy2xy^2. To do this, multiply xx by (y2/y2)(y^2/y^2) to get (xy2)/(xy2)(xy^2)/(xy^2).
  3. Combine Fractions: Combine the two fractions over the common denominator.\newlineNow we have (xy2)/(xy2)+(1)/(xy2)(xy^2)/(xy^2) + (1)/(xy^2), which can be combined into a single fraction as (xy2+1)/(xy2)(xy^2 + 1)/(xy^2).
  4. Check for Simplification: Check if the fraction can be simplified further.\newlineThe numerator xy2+1xy^2 + 1 and the denominator xy2xy^2 have no common factors other than 11, so the fraction is already in its simplest form.

More problems from Roots of rational numbers

Question
Find the real-number root. \newline16\sqrt{16}\newline\newlineWrite your answer in simplified form. \newline____\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root. \newline916\sqrt{\frac{9}{16}}\newlineSimplify your answer and write it as a decimal or as a proper or improper fraction.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root using a calculator. Round your answer to the nearest thousandth. \newline`\sqrt{957} `\newline _______
Get tutor helpright-arrow

Posted 9 months ago

Question
Multiply. Write your answer in simplest form. \newline273×42\sqrt{273} \times \sqrt{42}\newline______
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify. \newline634\sqrt{\frac{63}{4}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify. Rationalize the denominator.\newline−49−2\frac{-4}{9 - \sqrt{2}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for z z .\newline16=z 16 = \sqrt{z} \newlinez= z = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression. 14x14 \sqrt{14x^{14}} Write your answer in the form A A , B \sqrt{B} , or AB A\sqrt{B} , where A A and B B are constants or expressions in x x . Use at most one radical in your answer, and at most one absolute value symbol in your expression for A A . ______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression.\newline12x12\sqrt{12x^{12}}\newlineWrite your answer in the form AA, B\sqrt{B}, or ABA\sqrt{B}, where AA and BB are constants or expressions in xx. Use at most one radical in your answer, and at most one absolute value symbol in your expression for AA.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 9 months ago

Question
What is the value of sin⁡(5π6)? \sin \left(\frac{5 \pi}{6}\right) ? \newlineChoose 11 answer:\newline(A) −32 -\frac{\sqrt{3}}{2} \newline(B) −22 -\frac{\sqrt{2}}{2} \newline(C) 12 \frac{1}{2} \newline(D) 150150
Get tutor helpright-arrow

Posted 10 months ago

View all (169)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant