Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

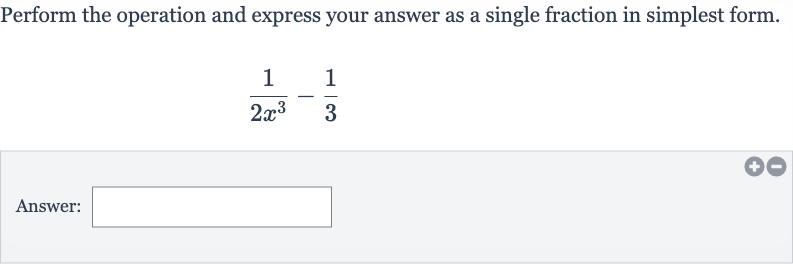
$Perform the operation and express your answer as a single fraction in simplest form. (1)/(2x^(3))-(1)/(3) Answer:$

Perform the operation and express your answer as a single fraction in simplest form. $\newline$ $\frac{1}{2 x^{3}}-\frac{1}{3}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Roots of rational numbers

Full solution

Q. Perform the operation and express your answer as a single fraction in simplest form.

\newline

\frac{1}{2 x^{3}}-\frac{1}{3}

\newline

Answer:

Identify LCD: Identify the least common denominator (LCD) for the two fractions. $\newline$ The LCD for the fractions $(\frac{1}{2x^{3}})$ and $(\frac{1}{3})$ is $6x^{3}$ because $6x^{3}$ is the smallest number that both denominators $(2x^{3}$ and $3)$ will divide into evenly.
Rewrite fractions: Rewrite each fraction with the LCD as the new denominator. $\newline$ For the first fraction, $(1)/(2x^{3})$ , we multiply the numerator and denominator by $3$ to get $(3)/(6x^{3})$ . $\newline$ For the second fraction, $(1)/(3)$ , we multiply the numerator and denominator by $2x^{3}$ to get $(2x^{3})/(6x^{3})$ .
Perform subtraction: Perform the subtraction of the two fractions. $\newline$ Now that both fractions have the same denominator, we can subtract their numerators: $\newline$ $(\frac{3}{6x^{3}}) - (\frac{2x^{3}}{6x^{3}}) = \frac{3 - 2x^{3}}{6x^{3}}$
Simplify fraction: Simplify the resulting fraction if possible. $\newline$ The fraction $(3 - 2x^{3})/(6x^{3})$ is already in its simplest form because the numerator and the denominator do not have any common factors other than $1$ .

More problems from Roots of rational numbers

Question

Find the real-number root.

\newline

\sqrt{16}

\newline

\newline

Write your answer in simplified form.

\newline

\_\_\_\_

Posted 9 months ago

Question

Find the real-number root.

\newline

\sqrt{\frac{9}{16}}

\newline

Simplify your answer and write it as a decimal or as a proper or improper fraction.

\newline

\_\_\_\_\_

Posted 9 months ago

Question

Find the real-number root using a calculator. Round your answer to the nearest thousandth.

\newline

\newline

Posted 9 months ago

Question

Multiply. Write your answer in simplest form.

\newline

\sqrt{273} \times \sqrt{42}

\newline

Posted 7 months ago

Question

\newline

\sqrt{\frac{63}{4}}

\newline

Posted 9 months ago

Question

Simplify. Rationalize the denominator.

\newline

\frac{-4}{9 - \sqrt{2}}

\newline

Posted 9 months ago

Question

z

\newline

16 = \sqrt{z}

\newline

z =

Posted 9 months ago

Question

Simplify the radical expression.

\sqrt{14x^{14}}

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

Posted 9 months ago

Question

Simplify the radical expression.

\newline

\sqrt{12x^{12}}

\newline

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

\newline

\underline{\hspace{3cm}}

Posted 9 months ago

Question

What is the value of

\sin \left(\frac{5 \pi}{6}\right) ?

\newline

1

\newline

-\frac{\sqrt{3}}{2}

\newline

-\frac{\sqrt{2}}{2}

\newline

\frac{1}{2}

\newline

150

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant