Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

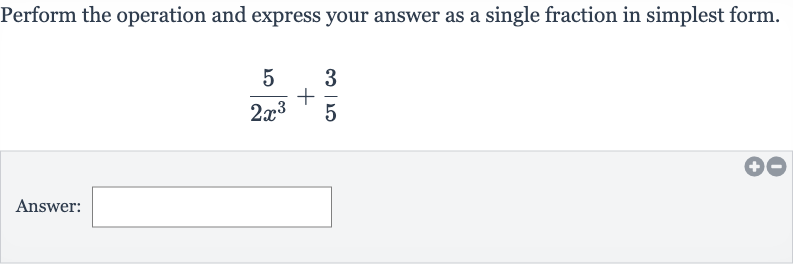
$Perform the operation and express your answer as a single fraction in simplest form. (5)/(2x^(3))+(3)/(5) Answer:$

Perform the operation and express your answer as a single fraction in simplest form. $\newline$ $\frac{5}{2 x^{3}}+\frac{3}{5}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Roots of rational numbers

Full solution

Q. Perform the operation and express your answer as a single fraction in simplest form.

\newline

\frac{5}{2 x^{3}}+\frac{3}{5}

\newline

Answer:

Find Common Denominator: To add the fractions $(5)/(2x^{3})$ and $(3)/(5)$ , we need to find a common denominator. The denominators are currently $2x^3$ and $5$ . The least common denominator (LCD) is the product of the distinct prime factors of each denominator, which in this case is $2x^3 \times 5$ .
Express Fractions: Now we need to express each fraction with the common denominator of $10x^3$ . To do this, we multiply the numerator and denominator of each fraction by the factor needed to reach the common denominator. For the first fraction, $\frac{5}{2x^{3}}$ , we multiply by $\frac{5}{5}$ to get $\frac{25}{10x^{3}}$ . For the second fraction, $\frac{3}{5}$ , we multiply by $\frac{2x^3}{2x^3}$ to get $\frac{6x^3}{10x^{3}}$ .
Add Fractions: Next, we add the two fractions with the common denominator: $(\frac{25}{10x^{3}}) + (\frac{6x^3}{10x^{3}})$ . This gives us a single fraction: $(\frac{25 + 6x^3}{10x^{3}})$ .
Simplify Final Answer: The fraction $(25 + 6x^3)/(10x^{3})$ is already in simplest form because the numerator and denominator have no common factors other than $1$ . Therefore, this is our final answer.

More problems from Roots of rational numbers

Question

Find the real-number root.

\newline

\sqrt{16}

\newline

\newline

Write your answer in simplified form.

\newline

\_\_\_\_

Posted 9 months ago

Question

Find the real-number root.

\newline

\sqrt{\frac{9}{16}}

\newline

Simplify your answer and write it as a decimal or as a proper or improper fraction.

\newline

\_\_\_\_\_

Posted 9 months ago

Question

Find the real-number root using a calculator. Round your answer to the nearest thousandth.

\newline

\newline

Posted 9 months ago

Question

Multiply. Write your answer in simplest form.

\newline

\sqrt{273} \times \sqrt{42}

\newline

Posted 7 months ago

Question

\newline

\sqrt{\frac{63}{4}}

\newline

Posted 9 months ago

Question

Simplify. Rationalize the denominator.

\newline

\frac{-4}{9 - \sqrt{2}}

\newline

Posted 9 months ago

Question

z

\newline

16 = \sqrt{z}

\newline

z =

Posted 9 months ago

Question

Simplify the radical expression.

\sqrt{14x^{14}}

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

Posted 9 months ago

Question

Simplify the radical expression.

\newline

\sqrt{12x^{12}}

\newline

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

\newline

\underline{\hspace{3cm}}

Posted 9 months ago

Question

What is the value of

\sin \left(\frac{5 \pi}{6}\right) ?

\newline

1

\newline

-\frac{\sqrt{3}}{2}

\newline

-\frac{\sqrt{2}}{2}

\newline

\frac{1}{2}

\newline

150

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant