Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

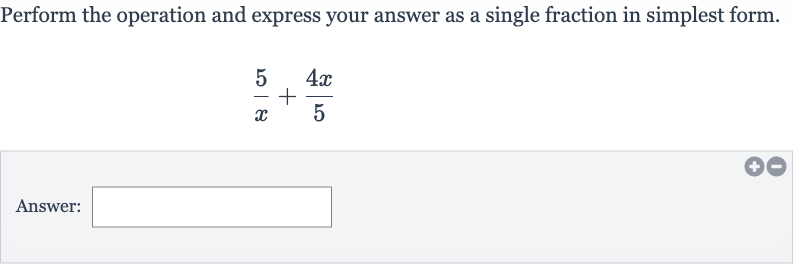
Perform the operation and express your answer as a single fraction in simplest form. (5)/(x)+(4x)/(5) Answer:

Perform the operation and express your answer as a single fraction in simplest form.\newline5x+4x5 \frac{5}{x}+\frac{4 x}{5} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Roots of rational numbersright chevron icon

Full solution

Q. Perform the operation and express your answer as a single fraction in simplest form.\newline5x+4x5 \frac{5}{x}+\frac{4 x}{5} \newlineAnswer:
  1. Identify Common Denominator: Identify a common denominator for the two fractions.\newlineThe common denominator for (5x)(\frac{5}{x}) and (4x5)(\frac{4x}{5}) is 5x5x.
  2. Rewrite with Common Denominator: Rewrite each fraction with the common denominator.\newline(5)/(x)(5)/(x) becomes (5×5)/(x×5)=(25)/(5x)(5\times5)/(x\times5) = (25)/(5x)\newline(4x)/(5)(4x)/(5) becomes (4x×x)/(5×x)=(4x2)/(5x)(4x\times x)/(5\times x) = (4x^2)/(5x)
  3. Add Fractions: Add the two fractions with the common denominator.\newline(255x)+(4x25x)=(25+4x25x)(\frac{25}{5x}) + (\frac{4x^2}{5x}) = (\frac{25 + 4x^2}{5x})
  4. Simplify Numerator: Simplify the numerator if possible.\newlineThe numerator 25+4x225 + 4x^2 cannot be factored or simplified further.
  5. Final Answer: Write the final answer as a single fraction in simplest form.\newlineThe sum of the two fractions is (25+4x25x)(\frac{25 + 4x^2}{5x}).

More problems from Roots of rational numbers

Question
Find the real-number root. \newline16\sqrt{16}\newline\newlineWrite your answer in simplified form. \newline____\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root. \newline916\sqrt{\frac{9}{16}}\newlineSimplify your answer and write it as a decimal or as a proper or improper fraction.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root using a calculator. Round your answer to the nearest thousandth. \newline`\sqrt{957} `\newline _______
Get tutor helpright-arrow

Posted 9 months ago

Question
Multiply. Write your answer in simplest form. \newline273×42\sqrt{273} \times \sqrt{42}\newline______
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify. \newline634\sqrt{\frac{63}{4}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify. Rationalize the denominator.\newline−49−2\frac{-4}{9 - \sqrt{2}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for z z .\newline16=z 16 = \sqrt{z} \newlinez= z = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression. 14x14 \sqrt{14x^{14}} Write your answer in the form A A , B \sqrt{B} , or AB A\sqrt{B} , where A A and B B are constants or expressions in x x . Use at most one radical in your answer, and at most one absolute value symbol in your expression for A A . ______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression.\newline12x12\sqrt{12x^{12}}\newlineWrite your answer in the form AA, B\sqrt{B}, or ABA\sqrt{B}, where AA and BB are constants or expressions in xx. Use at most one radical in your answer, and at most one absolute value symbol in your expression for AA.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 9 months ago

Question
What is the value of sin⁡(5π6)? \sin \left(\frac{5 \pi}{6}\right) ? \newlineChoose 11 answer:\newline(A) −32 -\frac{\sqrt{3}}{2} \newline(B) −22 -\frac{\sqrt{2}}{2} \newline(C) 12 \frac{1}{2} \newline(D) 150150
Get tutor helpright-arrow

Posted 10 months ago

View all (169)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant