Full solution
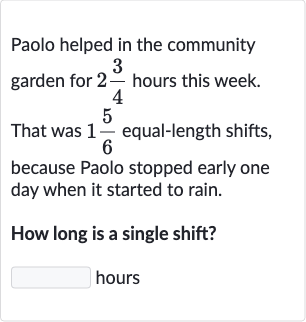
Q. Paolo helped in the community garden for hours this week. That was equal-length shifts, because Paolo stopped early one day when it started to rain. How long is a single shift? hours
- Convert to Improper Fraction: Paolo worked for hours this week, which is hours.Convert mixed number to improper fraction: hours.
- Convert Shifts to Improper Fraction: He worked shifts, which is shifts.Convert mixed number to improper fraction: shifts.
- Find Length of One Shift: To find the length of one shift, divide the total hours worked by the number of shifts.So, hours shifts = shifts.
- Simplify Expression: Simplify the expression by canceling out the common factor of ..
- Simplify to Lowest Terms: Simplify to its lowest terms. or hours per shift.
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help