Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

P(x)=x^(4)-3x^(2)+kx-2
where
k is an unknown integer.

P(x) divided by
(x-2) has a remainder of 10 .
What is the value of
k ?

k=

$P(x)=x^{4}-3 x^{2}+k x-2$ $\newline$ where $k$ is an unknown integer. $\newline$ $P(x)$ divided by $(x-2)$ has a remainder of $10$ . $\newline$ What is the value of $k$ ? $\newline$ $k=$

View step-by-step help

View step-by-step help

Evaluate recursive formulas for sequences

Full solution

Q.

P(x)=x^{4}-3 x^{2}+k x-2

\newline

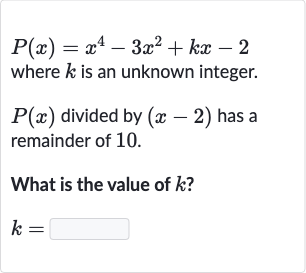
where

k

is an unknown integer.

\newline

P(x)

divided by

(x-2)

has a remainder of

10

.

\newline

What is the value of

k

?

\newline

k=

Apply Remainder Theorem: To find the value of $k$ , we will use the Remainder Theorem, which states that if a polynomial $P(x)$ is divided by $(x - c)$ , the remainder is $P(c)$ . In this case, $c$ is $2$ because we are dividing by $(x - 2)$ , and the remainder is given as $10$ . So we need to find $P(2)$ .
Substitute $x = 2$ : We substitute $x = 2$ into the polynomial $P(x) = x^4 - 3x^2 + kx - 2$ .
$P(2) = (2)^4 - 3(2)^2 + k(2) - 2$
$P(2) = 16 - 3(4) + 2k - 2$
$P(2) = 16 - 12 + 2k - 2$
Simplify $P(2)$ : Now we simplify the expression for $P(2)$ .
$P(2) = 16 - 12 - 2 + 2k$
$P(2) = 2 + 2k$
Set $P(2) = 10$ : Since the remainder when $P(x)$ is divided by $(x - 2)$ is $10$ , we set $P(2)$ equal to $10$ . $\newline$ $2 + 2k = 10$
Solve for k: We solve for k by subtracting $2$ from both sides of the equation. $\newline$ $2k = 10 - 2$ $\newline$ $2k = 8$
Final Value of k: Now we divide both sides by $2$ to find the value of k. $\newline$ k = $\frac{8}{2}$ $\newline$ k = $4$

More problems from Evaluate recursive formulas for sequences

Question

Find the sum of the finite arithmetic series.

\sum_{n=1}^{10} (7n+4)

\newline

Posted 3 months ago

Question

Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`

Posted 2 months ago

Question

Type the missing number in this sequence: `2, 4 8`, ______,`32`

Posted 2 months ago

Question

What kind of sequence is this?

2, 10, 50, 250, \ldots

Choices:Choices:

\newline

\text{[A]arithmetic}

\newline

\text{[B]geometric}

\newline

\text{[C]both}

\newline

\text{[D]neither}

Posted 2 months ago

Question

What is the missing number in this pattern?

1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_

Posted 2 months ago

Question

Classify the series.

\sum_{n = 0}^{12} (n + 2)^3

\newline

\newline

\text{[A]arithmetic}

\newline

\text{[B]geometric}

\newline

\text{[C]both}

\newline

\text{[D]neither}

Posted 2 months ago

Question

Find the first three partial sums of the series.

\newline

1 + 6 + 11 + 16 + 21 + 26 + \cdots

\newline

Write your answers as integers or fractions in simplest form.

\newline

S_1 =

\newline

S_2 =

\newline

S_3 =

Posted 2 months ago

Question

Find the third partial sum of the series.

\newline

3 + 9 + 15 + 21 + 27 + 33 + \cdots

\newline

Write your answer as an integer or a fraction in simplest form.

\newline

S_3 =

Posted 2 months ago

Question

Find the first three partial sums of the series.

\newline

1 + 7 + 13 + 19 + 25 + 31 + \cdots

\newline

Write your answers as integers or fractions in simplest form.

\newline

S_1 =

\newline

S_2 =

\newline

S_3 =

Posted 3 months ago

Question

Does the infinite geometric series converge or diverge?

\newline

1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots

\newline

\newline

\text{[A]converge}

\newline

\text{[B]diverge}

Posted 2 months ago