Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

P(x)=2x^(4)-x^(3)+2x^(2)-k
where
k is an unknown integer.

P(x) divided by
(x+1) has a remainder of 2 .
What is the value of
k ?

k=

$P(x)=2 x^{4}-x^{3}+2 x^{2}-k$ $\newline$ where $k$ is an unknown integer. $\newline$ $P(x)$ divided by $(x+1)$ has a remainder of $2$ . $\newline$ What is the value of $k$ ? $\newline$ $k=$

View step-by-step help

View step-by-step help

Evaluate recursive formulas for sequences

Full solution

Q.

P(x)=2 x^{4}-x^{3}+2 x^{2}-k

\newline

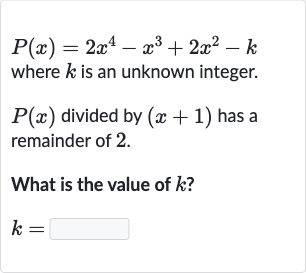
where

k

is an unknown integer.

\newline

P(x)

divided by

(x+1)

has a remainder of

2

.

\newline

What is the value of

k

?

\newline

k=

Apply Remainder Theorem: To find the value of $k$ , we will use the Remainder Theorem, which states that if a polynomial $P(x)$ is divided by $(x - c)$ , the remainder is $P(c)$ . Since we are dividing by $(x + 1)$ , we will find $P(-1)$ .
Substitute $x = -1$ : Substitute $x = -1$ into the polynomial $P(x) = 2x^4 - x^3 + 2x^2 - k$ . $\newline$ $P(-1) = 2(-1)^4 - (-1)^3 + 2(-1)^2 - k$ $\newline$ $P(-1) = 2(1) - (-1) + 2(1) - k$ $\newline$ $P(-1) = 2 + 1 + 2 - k$ $\newline$ $P(-1) = 5 - k$
Set $P(-1)$ equal to $2$ : According to the problem, the remainder when $P(x)$ is divided by $(x + 1)$ is $2$ . Therefore, we set $P(-1)$ equal to $2$ . $\newline$ $5 - k = 2$
Solve for k: Solve for k. $\newline$ $5 - k = 2$ $\newline$ $k = 5 - 2$ $\newline$ $k = 3$

More problems from Evaluate recursive formulas for sequences

Question

Find the sum of the finite arithmetic series.

\sum_{n=1}^{10} (7n+4)

\newline

Posted 2 months ago

Question

Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`

Posted 1 month ago

Question

Type the missing number in this sequence: `2, 4 8`, ______,`32`

Posted 2 months ago

Question

What kind of sequence is this?

2, 10, 50, 250, \ldots

Choices:Choices:

\newline

\text{[A]arithmetic}

\newline

\text{[B]geometric}

\newline

\text{[C]both}

\newline

\text{[D]neither}

Posted 2 months ago

Question

What is the missing number in this pattern?

1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_

Posted 1 month ago

Question

Classify the series.

\sum_{n = 0}^{12} (n + 2)^3

\newline

\newline

\text{[A]arithmetic}

\newline

\text{[B]geometric}

\newline

\text{[C]both}

\newline

\text{[D]neither}

Posted 1 month ago

Question

Find the first three partial sums of the series.

\newline

1 + 6 + 11 + 16 + 21 + 26 + \cdots

\newline

Write your answers as integers or fractions in simplest form.

\newline

S_1 =

\newline

S_2 =

\newline

S_3 =

Posted 1 month ago

Question

Find the third partial sum of the series.

\newline

3 + 9 + 15 + 21 + 27 + 33 + \cdots

\newline

Write your answer as an integer or a fraction in simplest form.

\newline

S_3 =

Posted 1 month ago

Question

Find the first three partial sums of the series.

\newline

1 + 7 + 13 + 19 + 25 + 31 + \cdots

\newline

Write your answers as integers or fractions in simplest form.

\newline

S_1 =

\newline

S_2 =

\newline

S_3 =

Posted 2 months ago

Question

Does the infinite geometric series converge or diverge?

\newline

1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots

\newline

\newline

\text{[A]converge}

\newline

\text{[B]diverge}

Posted 1 month ago