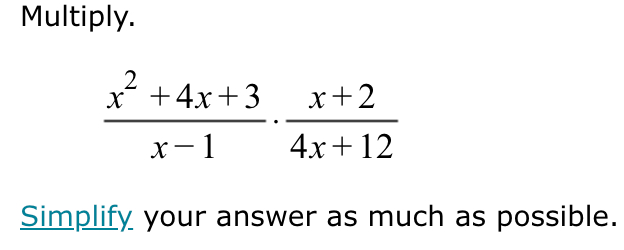Multiply. (x^(2)+4x+3)/(x-1)*(x+2)/(4x+12) Simplify your answer as much as possible.

Question

Multiply.  (x^(2)+4x+3)/(x-1)*(x+2)/(4x+12) Simplify your answer as much as possible.

Bytelearn · Accepted Answer

Identify Expressions: Identify the expressions to be multiplied. We have two fractions to multiply: (x^2 + 4x + 3)/(x - 1) and (x + 2)/(4x + 12).Factor Numerators and Denominators: Factor the numerators and denominators where possible. The numerator x^2 + 4x + 3 can be factored into (x + 1)(x + 3). The denominator 4x + 12 can be factored into 4(x + 3).Rewrite with Factored Terms: Rewrite the original expression with the factored terms. The expression becomes ((x + 1)(x + 3))/(x - 1) * (x + 2)/4(x + 3).Cancel Common Factors: Cancel out common factors from the numerator and denominator. The (x + 3) term is present in both a numerator and a denominator, so it can be canceled out. The expression simplifies to ((x + 1))/(x - 1) * (x + 2)/4.Multiply Numerators and Denominators: Multiply the remaining numerators and denominators. Multiply the numerators: (x + 1) * (x + 2). Multiply the denominators: (x - 1) * 4.Perform Numerator Multiplication: Perform the multiplication in the numerators. (x + 1) * (x + 2) equals x^2 + 2x + x + 2, which simplifies to x^2 + 3x + 2.Perform Denominator Multiplication: Perform the multiplication in the denominators. (x - 1) * 4 equals 4x - 4.Write Final Expression: Write the final simplified expression. The final simplified expression is (x^2 + 3x + 2)/(4x - 4).

Multiply. $\newline$ $\frac{x^{2}+4 x+3}{x-1} \cdot \frac{x+2}{4 x+12}$ $\newline$ Simplify your answer as much as possible.

Full solution

More problems from Multiply and divide rational expressions

Related topics

Multiply.\newlinex2+4x+3x−1⋅x+24x+12 \frac{x^{2}+4 x+3}{x-1} \cdot \frac{x+2}{4 x+12} x−1x2+4x+3​⋅4x+12x+2​\newlineSimplify your answer as much as possible.

Multiply. $\newline$ $\frac{x^{2}+4 x+3}{x-1} \cdot \frac{x+2}{4 x+12}$ $\newline$ Simplify your answer as much as possible.