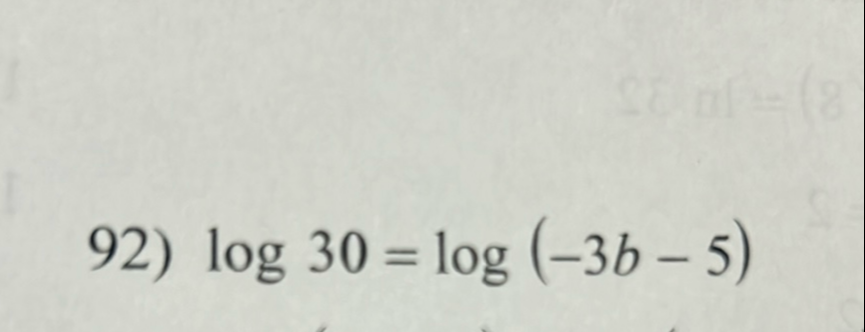AI tutor
Full solution
Q. )
- Understand Equation: Understand the given logarithmic equation.The equation implies that the logarithmic expressions on both sides of the equation are equal. Therefore, the arguments of the logarithms must be equal.
- Set Arguments Equal: Set the arguments of the logarithms equal to each other.Since the logs are equal and have the same base, we can set their arguments equal to each other:
- Solve for b: Solve for b.To find the value of , we need to isolate on one side of the equation. We will add to both sides and then divide by .