Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_((1)/(5))5=

$\log _{\frac{1}{5}} 5=$

View step-by-step help

View step-by-step help

Add and subtract rational numbers

Full solution

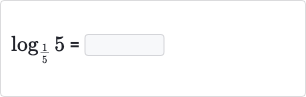
Q.

\log _{\frac{1}{5}} 5=

Identify Base and Argument: Identify the base of the logarithm and the argument. The base is $(\frac{1}{5})$ and the argument is $5$ .
Recall Logarithm Definition: Recall the definition of a logarithm: $\log_{\text{base}}(\text{argument}) = \text{exponent}$ means that $\text{base}^{\text{exponent}} = \text{argument}$ .
Determine Exponent: Determine the exponent that makes $(\frac{1}{5})$ raised to that power equal to $5$ . Since $(\frac{1}{5})^{-1} = 5$ , the exponent we are looking for is $-1$ .
Conclude Solution: Conclude that $\log_{\left(\frac{1}{5}\right)}5 = -1$ because $\left(\frac{1}{5}\right)^{-1} = 5$ .

More problems from Add and subtract rational numbers

Question

\newline

\frac{1}{3} \div \frac{1}{4}=

Posted 4 months ago

Question

-5-15+4+15

Posted 3 months ago

Question

\frac{15}{x}-4=\frac{2}{x}

Posted 3 months ago

Question

Let us write the multiplication table of

1

\newline

\begin{array}{cc}\(\newline1\times1=1 & 1\times2=2 (\newline\)1\times6=6 & 1\times7=7

\newline

Posted 3 months ago

Question

10 + 20

Posted 2 months ago

Question

A waitress sold

10

ribeye steak dinners and

10

grilled salmon dinners, totaling

\$584.01

on a particular day. Another day she sold

11

ribeye steak dinners and

5

grilled salmon dinners, totaling

\$584.45

. How much did each type of dinner cost?

Posted 2 months ago

Question

(1)/(6)

of a packet of flour to make some muffins and

(1)/(4)

of it to make a cake She used the remainder to make some cupcakes. She used

(1)/(12)

of the packet of flour or each cupcake. How many cupcakes did she make?

Posted 2 months ago

Question

375/5

Posted 2 months ago

Question

\newline

23

\newline

24

\newline

25

\newline

26

\newline

Solve by the method of your choice.

\newline

Twenty-two people purchase raffle tickets. Three winning tickets are selected at random. If first prize is \$\(1000\), second prize is \$\(500\), and third prize is \$\(100\), in how many different ways can the prizes be awarded?\(\newline\)There are \(\square\) different ways in which the prizes can be awarded.\(\newline\)(Simplify your answer.)

Posted 2 months ago

Question

C=0.25(L-1200)

\newline

C

, in dollars, to the holder of a liability insurance account given a total liability of

L

dollars is given by the equation as long as

C

is positive. By how many dollars does the liability increase when the cost to the holder increases by

1

\newline

\square

Posted 18 hours ago