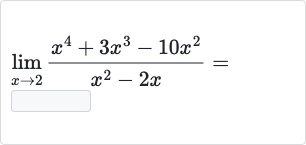lim_(x rarr2)(x^(4)+3x^(3)-10x^(2))/(x^(2)-2x)=

Question

Bytelearn · Accepted Answer

Identify Function & Point: Identify the function and the point at which we need to find the limit. We are given the function (x^4 + 3x^3 - 10x^2) / (x^2 - 2x) and we need to find the limit as x approaches 2.Direct Substitution: Try direct substitution of x = 2 into the function to see if the limit can be found this way. Substitute x = 2 into the function: ((2)^4 + 3(2)^3 - 10(2)^2) / ((2)^2 - 2(2)) = (16 + 24 - 40) / (4 - 4) = 0 / 0 We get an indeterminate form 0/0, which means we cannot find the limit by direct substitution.Factor Numerator & Denominator: Factor the numerator and denominator to simplify the expression. Factor x^2 out of the numerator and x out of the denominator: (x^2(x^2 + 3x - 10)) / (x(x - 2)) Now, factor the quadratic x^2 + 3x - 10: (x^2(x + 5)(x - 2)) / (x(x - 2))Cancel Common Factors: Cancel out the common factors in the numerator and the denominator. Cancel the common factor (x - 2): (x^2(x + 5)) / xSimplify Expression: Simplify the expression further by canceling out any more common factors. Cancel the common factor x: (x(x + 5))Try Direct Substitution Again: Now that the expression is simplified, try direct substitution again. Substitute x = 2 into the simplified function: (2(2 + 5)) = (2 * 7) = 14

$\lim _{x \rightarrow 2} \frac{x^{4}+3 x^{3}-10 x^{2}}{x^{2}-2 x}=$

Full solution

More problems from Negative Exponents

Related topics

lim⁡x→2x4+3x3−10x2x2−2x= \lim _{x \rightarrow 2} \frac{x^{4}+3 x^{3}-10 x^{2}}{x^{2}-2 x}= limx→2​x2−2xx4+3x3−10x2​=

$\lim _{x \rightarrow 2} \frac{x^{4}+3 x^{3}-10 x^{2}}{x^{2}-2 x}=$