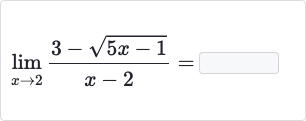lim_(x rarr2)(3-sqrt(5x-1))/(x-2)=

Question

Bytelearn · Accepted Answer

Identify the form: Identify the form of the limit. Substitute x = 2 into the function to see if the limit can be directly calculated. lim_(x -> 2)(3 - sqrt(5x - 1)) / (x - 2) = (3 - sqrt(5*2 - 1)) / (2 - 2) = (3 - sqrt(9)) / 0 = (3 - 3) / 0 = 0/0 This is an indeterminate form, so we cannot directly calculate the limit.Substitute and check: Apply L'Hôpital's Rule. Since we have an indeterminate form of 0/0, we can apply L'Hôpital's Rule, which states that if the limit as x approaches a of f(x)/g(x) is 0/0 or ∞/∞, then the limit is the same as the limit of the derivatives of the numerator and the denominator. So, we need to find the derivatives of the numerator and the denominator.Apply L'Hôpital's Rule: Differentiate the numerator and the denominator. The derivative of the numerator 3 - sqrt(5x - 1) with respect to x is: d/dx[3 - sqrt(5x - 1)] = d/dx[3] - d/dx[sqrt(5x - 1)] = 0 - (1/2)(5x - 1)^(-1/2) * 5 = -5/(2sqrt(5x - 1)) The derivative of the denominator x - 2 with respect to x is: d/dx[x - 2] = 1Differentiate numerator and denominator: Apply L'Hôpital's Rule using the derivatives. Now we take the limit of the derivatives: lim_(x -> 2)(-5/(2sqrt(5x - 1))) / (1) = -5/(2sqrt(5*2 - 1))Apply Rule with derivatives: Calculate the limit of the derivatives. Substitute x = 2 into the derivative of the numerator: lim_(x -> 2)(-5/(2sqrt(5x - 1))) = -5/(2sqrt(5*2 - 1)) = -5/(2sqrt(9)) = -5/(2*3) = -5/6

$\lim _{x \rightarrow 2} \frac{3-\sqrt{5 x-1}}{x-2}=$

Full solution

More problems from Identify equivalent linear expressions I

Related topics

lim⁡x→23−5x−1x−2= \lim _{x \rightarrow 2} \frac{3-\sqrt{5 x-1}}{x-2}= limx→2​x−23−5x−1​​=

$\lim _{x \rightarrow 2} \frac{3-\sqrt{5 x-1}}{x-2}=$