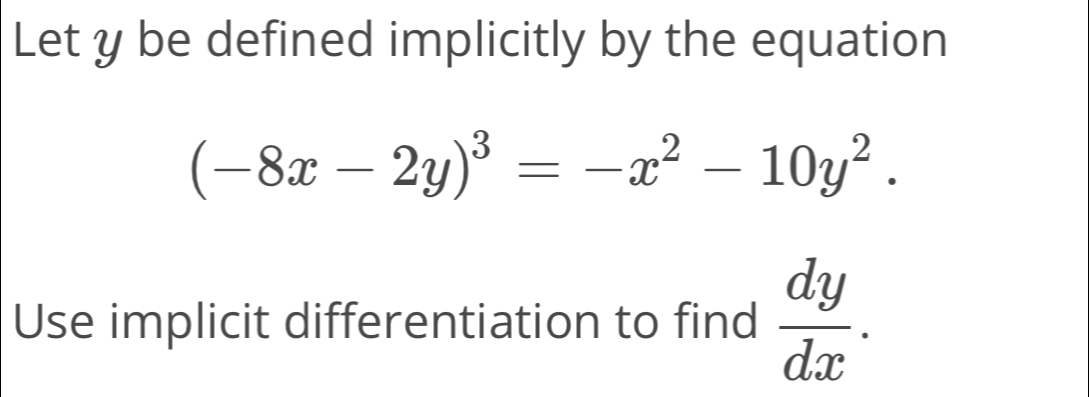Let y be defined implicitly by the equation (-8x-2y)^(3)=-x^(2)-10y^(2). Use implicit differentiation to find (dy)/(dx).

Question

Let  y be defined implicitly by the equation  (-8x-2y)^(3)=-x^(2)-10y^(2). Use implicit differentiation to find  (dy)/(dx).

Bytelearn · Accepted Answer

Differentiate with respect to x: Differentiate both sides of the equation with respect to x. We have the equation (-8x-2y)^(3)=-x^(2)-10y^(2). We will apply the chain rule to the left side and the power rule to the right side. Remember that when differentiating y with respect to x, we treat y as a function of x and use the chain rule to include (dy)/(dx).Apply chain rule to left side: Apply the chain rule to the left side of the equation. The derivative of (-8x-2y)^(3) with respect to x is 3*(-8x-2y)^(2)*(-8 - 2*(dy)/(dx)), where we have multiplied by the derivative of the inside function (-8x-2y), which is -8 for the x part and -2*(dy)/(dx) for the y part because y is a function of x.Apply power rule to right side: Apply the power rule and chain rule to the right side of the equation. The derivative of -x^(2) with respect to x is -2x, and the derivative of -10y^(2) with respect to x is -20y*(dy)/(dx), where we have multiplied by (dy)/(dx) because y is a function of x.Write down differentiated equation: Write down the differentiated equation. We now have 3*(-8x-2y)^(2)*(-8 - 2*(dy)/(dx)) = -2x - 20y*(dy)/(dx).Solve for dy/dx: Solve for (dy)/(dx). To solve for (dy)/(dx), we need to collect all terms containing (dy)/(dx) on one side and the rest on the other side. We get: 3*(-8x-2y)^(2)*(-2*(dy)/(dx)) - 20y*(dy)/(dx) = -2x - 3*(-8x-2y)^(2)*(-8).Factor out dy/dx: Factor out (dy)/(dx) from the left side of the equation. (dy)/(dx) * (3*(-8x-2y)^(2)*(-2) - 20y) = -2x - 3*(-8x-2y)^(2)*(-8).Isolate dy/dx: Isolate (dy)/(dx). (dy)/(dx) = (-2x - 3*(-8x-2y)^(2)*(-8)) / (3*(-8x-2y)^(2)*(-2) - 20y).Simplify expression for dy/dx: Simplify the expression for (dy)/(dx) if possible. We can leave the expression as it is because it does not simplify easily. So, the final answer is: (dy)/(dx) = (-2x + 3*8*(-8x-2y)^(2)) / (-6*(-8x-2y)^(2) - 20y).

Let $y$ be defined implicitly by the equation $\newline$ $(-8 x-2 y)^{3}=-x^{2}-10 y^{2} .$ $\newline$ Use implicit differentiation to find $\frac{d y}{d x}$ .

Full solution

More problems from Simplify expressions using trigonometric identities

Related topics

Let y y y be defined implicitly by the equation\newline(−8x−2y)3=−x2−10y2. (-8 x-2 y)^{3}=-x^{2}-10 y^{2} . (−8x−2y)3=−x2−10y2.\newlineUse implicit differentiation to find dydx \frac{d y}{d x} dxdy​.

Let $y$ be defined implicitly by the equation $\newline$ $(-8 x-2 y)^{3}=-x^{2}-10 y^{2} .$ $\newline$ Use implicit differentiation to find $\frac{d y}{d x}$ .