Full solution
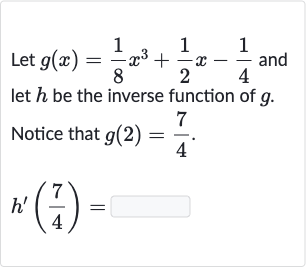
Q. Let and let be the inverse function of . Notice that .
- Find : First, we need to find the derivative of , which is . So let's differentiate .
- Calculate : .
- Use formula for inverse function derivative: Now, we know that , so we need to find to use in the formula for the derivative of the inverse function.
- Find or
- Plug in into formula: The formula for the derivative of the inverse function at a point is . We have and we need to find , but we already know that , so .
- Simplify expression: Now we plug in into the formula: .
- Simplify expression: Now we plug in into the formula: .Simplify the expression: .