Let $f(x)=2 \cos \left(\frac{\pi x}{4}\right)$ and $g(x)=x-6$ . $\newline$ Find the sum of the areas enclosed by the graphs of $f$ and $g$ between $x=4$ and $x=8$ . $\newline$ Use a graphing calculator and round your answer to three decimal places.

View step-by-step help

Home

Math Problems

Precalculus

Find the magnitude of a three-dimensional vector

Full solution

Q. Let

f(x)=2 \cos \left(\frac{\pi x}{4}\right)

and

g(x)=x-6

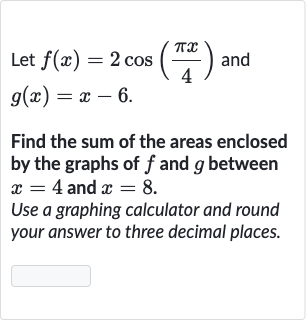
\newline

Find the sum of the areas enclosed by the graphs of

f

and

g

between

x=4

and

x=8

\newline

Use a graphing calculator and round your answer to three decimal places.

Calculate Total Area: To find the sum of the areas enclosed by the graphs of $f(x)$ and $g(x)$ between $x = 4$ and $x = 8$ , we need to calculate the definite integral of the absolute value of the difference between $f(x)$ and $g(x)$ over the interval $[4, 8]$ . This will give us the total area between the two curves.
Find Intersection Points: First, we need to find the points of intersection between $f(x)$ and $g(x)$ to determine if there are any within the interval $[4, 8]$ . This is done by setting $f(x)$ equal to $g(x)$ and solving for $x$ . $2\cos\left(\frac{\pi x}{4}\right) = x - 6$
Calculate Area $1$ : We will use a graphing calculator to solve the equation $2\cos\left(\frac{\pi x}{4}\right) = x - 6$ , as it cannot be solved algebraically. We are looking for solutions within the interval $[4, 8]$ .
Calculate Area $2$ : After using the graphing calculator, we find that the two functions intersect at $x = 6$ . This means we will need to calculate the area between the curves from $x = 4$ to $x = 6$ and from $x = 6$ to $x = 8$ separately.
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ . $\newline$ Area $_1$ = $\int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \, dx$
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ . $\newline$ Area $1$ = $\int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of Area $1$ by evaluating the definite integral. $\newline$ Area $1$ $\approx$ [integral value from the calculator]
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ .
$\text{Area1} = \int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of $\text{Area1}$ by evaluating the definite integral.
$\text{Area1} \approx [\text{integral value from the calculator}]$ For the interval $[6, 8]$ , $g(x)$ is above $f(x)$ . We calculate the area by integrating the difference $f(x)$ $2$ from $x = 6$ to $f(x)$ $4$ .
$f(x)$ $5$
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ .
$\text{Area1} = \int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of $\text{Area1}$ by evaluating the definite integral.
$\text{Area1} \approx [\text{integral value from the calculator}]$ For the interval $[6, 8]$ , $g(x)$ is above $f(x)$ . We calculate the area by integrating the difference $f(x)$ $2$ from $x = 6$ to $f(x)$ $4$ .
$f(x)$ $5$ Using the graphing calculator, we find the value of $f(x)$ $6$ by evaluating the definite integral.
$f(x)$ $7$
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ .
$\text{Area1} = \int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of $\text{Area1}$ by evaluating the definite integral.
$\text{Area1} \approx [\text{integral value from the calculator}]$ For the interval $[6, 8]$ , $g(x)$ is above $f(x)$ . We calculate the area by integrating the difference $f(x)$ $2$ from $x = 6$ to $f(x)$ $4$ .
$f(x)$ $5$ Using the graphing calculator, we find the value of $f(x)$ $6$ by evaluating the definite integral.
$f(x)$ $7$ The sum of the areas enclosed by the graphs of $f(x)$ and $g(x)$ between $x = 4$ and $f(x)$ $4$ is the sum of $\text{Area1}$ and $f(x)$ $6$ .
$g(x)$ $4$
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ .
$\text{Area1} = \int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of Area $1$ by evaluating the definite integral.
$\text{Area1} \approx [\text{integral value from the calculator}]$ For the interval $[6, 8]$ , $g(x)$ is above $f(x)$ . We calculate the area by integrating the difference $f(x)$ $1$ from $x = 6$ to $f(x)$ $3$ .
$f(x)$ $4$ Using the graphing calculator, we find the value of Area $2$ by evaluating the definite integral.
$f(x)$ $5$ The sum of the areas enclosed by the graphs of $f(x)$ and $g(x)$ between $x = 4$ and $f(x)$ $3$ is the sum of Area $1$ and Area $2$ .
$g(x)$ $0$ We add the values obtained from the graphing calculator for Area $1$ and Area $2$ to get the Total Area.
$g(x)$ $1$
Sum of Areas: For the interval $[4, 6]$ , $f(x)$ is above $g(x)$ . We calculate the area by integrating the difference $f(x) - g(x)$ from $x = 4$ to $x = 6$ .
$\text{Area1} = \int_{4}^{6} (2\cos(\frac{\pi x}{4}) - (x - 6)) \,dx$ Using the graphing calculator, we find the value of Area $1$ by evaluating the definite integral.
$\text{Area1} \approx [\text{integral value from the calculator}]$ For the interval $[6, 8]$ , $g(x)$ is above $f(x)$ . We calculate the area by integrating the difference $f(x)$ $1$ from $x = 6$ to $f(x)$ $3$ .
$f(x)$ $4$ Using the graphing calculator, we find the value of Area $2$ by evaluating the definite integral.
$f(x)$ $5$ The sum of the areas enclosed by the graphs of $f(x)$ and $g(x)$ between $x = 4$ and $f(x)$ $3$ is the sum of Area $1$ and Area $2$ .
$g(x)$ $0$ We add the values obtained from the graphing calculator for Area $1$ and Area $2$ to get the Total Area.
$g(x)$ $1$ After rounding the Total Area to three decimal places, we get the final answer.
$g(x)$ $2$