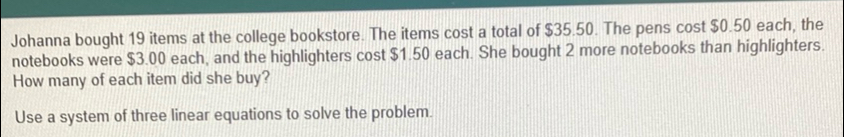
Johanna bought 19 items at the college bookstore. The items cost a total of $35.50. The pens cost $0.50 each, the notebooks were $3.00 each, and the highlighters cost $1.50 each. She bought 2 more notebooks than highlighters. How many of each item did she buy? Use a system of three linear equations to solve the problem.

Question

Johanna bought 19 items at the college bookstore. The items cost a total of  $35.50. The pens cost  $0.50 each, the notebooks were  $3.00 each, and the highlighters cost  $1.50 each. She bought 2 more notebooks than highlighters. How many of each item did she buy? Use a system of three linear equations to solve the problem.

Bytelearn · Accepted Answer

Define variables for items:  Define variables for each type of item Johanna bought: let p be the number of pens, n be the number of notebooks, and h be the number of highlighters. Johanna bought a total of 19 items. Set up total cost equation:  Set up the total cost equation using the prices given for each item. Pens cost $0.50 each, notebooks $3.00 each, and highlighters $1.50 each. The total cost of all items is $35.50. Express relationship between notebooks and highlighters:  Johanna bought 2 more notebooks than highlighters. This relationship can be expressed as an equation. Substitute equations to reduce variables:  Substitute the equation from step 3 into the equations from steps 1 and 2 to reduce the number of variables. Replace n with (h + 2) in both equations. Simplify equations:  Simplify the equations obtained in step 4. Further simplify and rearrange:  Further simplify and rearrange the equations. Solve for number of pens:  Solve one of the equations for p. From the first simplified equation, express p in terms of h. Substitute pen expression into equation:  Substitute the expression for p from step 6 into the second simplified equation. Distribute and combine terms:  Distribute and combine like terms in the equation from step 7. Solve for highlighters:  Solve for h.

Full solution

More problems from Solve a system of equations using elimination: word problems

Related topics