Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Item 23 of 27
Let the function
h be defined as
h(x)=c*2^(x-c)+k, where
c and
k are constants. If
h(c)=20, which of the following equations correctly expresses
c in terms of
k?

Let the function $h$ be defined as $h(x)=c \cdot 2^{x-c}+k$ , where $c$ and $k$ are constants. If $h(c)=20$ , which of the following equations correctly expresses $c$ in terms of $k ?$

View step-by-step help

View step-by-step help

Write a quadratic function from its x-intercepts and another point

Full solution

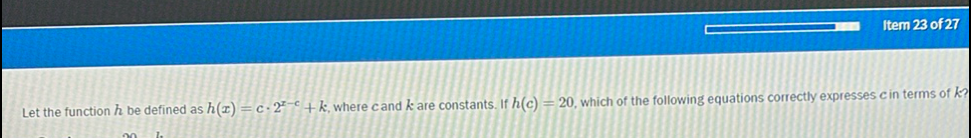
Q. Let the function

h

be defined as

h(x)=c \cdot 2^{x-c}+k

, where

c

and

k

are constants. If

h(c)=20

, which of the following equations correctly expresses

c

in terms of

k ?

Substitute $x$ with $c$ : We are given the function $h(x) = c \cdot 2^{(x-c)} + k$ and the condition $h(c) = 20$ . We need to find the value of $c$ in terms of $k$ . First, let's substitute $x$ with $c$ in the function $h(x)$ to apply the given condition $h(c) = 20$ . $c$ $0$
Simplify using exponents: Now, let's simplify the equation using the property of exponents that any number to the power of $0$ is $1$ .
$h(c) = c \cdot 2^0 + k$
$h(c) = c \cdot 1 + k$
$h(c) = c + k$
Substitute $h(c)$ with $20$ : We know that $h(c) = 20$ , so we can substitute $20$ for $h(c)$ in the equation. $\newline$ $20 = c + k$
Isolate $c$ in equation: To express $c$ in terms of $k$ , we need to isolate $c$ on one side of the equation. We do this by subtracting $k$ from both sides of the equation. $\newline$ $20 - k = c + k - k$ $\newline$ $20 - k = c$
Final expression for $c$ : We have now expressed $c$ in terms of $k$ . The equation is: $\newline$ $c = 20 - k$ $\newline$ This is the final answer.

More problems from Write a quadratic function from its x-intercepts and another point

Question

Solve by completing the square.

\newline

m^2 - 10m - 29 = 0

\newline

Write your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.

\newline

`m` = ____ or `m` = _____

Posted 8 months ago

Question

g(x)

g(x)

is the translation

5

f(x)=x^2

\newline

Write your answer in the form

a(x–h)^2+k

a

h

k

\newline

g(x)=

Posted 7 months ago

Question

What is the range of this quadratic function?

\newline

y = x^2 - 4x + 4

\newline

\newline

\left\{y \mid y \geq 2\right\}

\newline

\left\{y \mid y \leq 0\right\}

\newline

\left\{y \mid y \geq 0\right\}

\newline

\text{all real numbers}

Posted 5 months ago

Question

Write the equation of the parabola that passes through the points

(1,0)

(2,0)

(3,\text{–}16)

. Write your answer in the form

y = a(x – p)(x – q)

a

p

q

are integers, decimals, or simplified fractions.

\newline

Posted 5 months ago

Question

Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.

\newline

f^2 + 8f + \_\_\_\_\_

Posted 5 months ago

Question

h

\newline

h^2 + 39h = 0

\newline

\newline

Write each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.

\newline

h =

\newline

Posted 5 months ago

Question

Write a quadratic function with zeros

-9

-7

\newline

Write your answer using the variable

x

and in standard form with a leading coefficient of

1

\newline

f(x) = \_\_\_\_\_

Posted 5 months ago

Question

Find the equation of the axis of symmetry for the parabola

y = x^2

\newline

Simplify any numbers and write them as proper fractions, improper fractions, or integers.

\newline

\underline{\hspace{3cm}}

Posted 4 months ago

Question

g(x)

g(x)

is the translation

8

f(x) = x^2

\newline

Write your answer in the form

a(x – h)^2 + k

a

h

k

\newline

g(x) =

\newline

Posted 4 months ago

Question

x

\newline

x^2 = 1

\newline

\newline

Write your answer in simplified, rationalized form.

\newline

x =

x =

\newline

Posted 5 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant