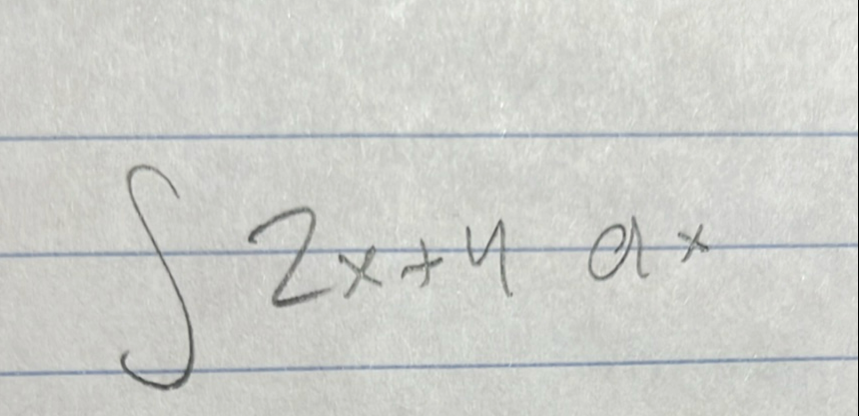AI tutor
Full solution
Q.
- Recognize the integral: Recognize the integral to be solved.We need to find the integral of the function with respect to . This is a basic indefinite integral involving a linear function.
- Apply power rule: Apply the power rule for integration to the term . The power rule for integration states that the integral of with respect to is , where is the constant of integration. For the term , which is to the power of , we apply the power rule.
- Calculate integral of : Calculate the integral of . The integral of with respect to is .
- Integrate constant term: Integrate the constant term . The integral of a constant with respect to is . Therefore, the integral of with respect to is .
- Combine results: Combine the results of Step and Step .The integral of with respect to is the sum of the integrals of and , which is , where is the constant of integration.
More problems from Sin, cos, and tan of special angles
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help