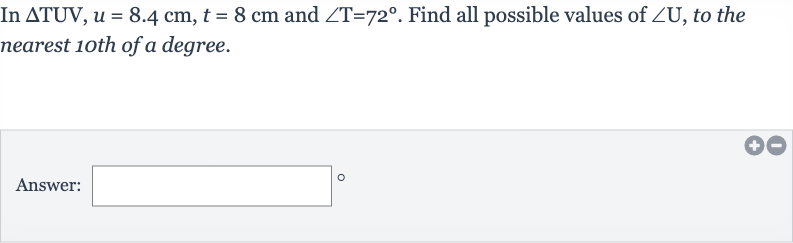
In /_\TUV,u=8.4cm,t=8cm and /_T=72^(@). Find all possible values of /_U, to the nearest 1oth of a degree. Answer:

Question

In  /_\TUV,u=8.4cm,t=8cm and  /_T=72^(@). Find all possible values of  /_U, to the nearest 1oth of a degree. Answer:

Bytelearn · Accepted Answer

Use Law of Cosines: Use the Law of Cosines to find the length of side v. The Law of Cosines states that for any triangle with sides a, b, and c, and the angle opposite side c being γ, the following equation holds: c^2 = a^2 + b^2 - 2ab*cos(γ). In this case, we want to find the length of side v, which is opposite the angle we know, /_T. We can set up the equation as follows: v^2 = t^2 + u^2 - 2*t*u*cos(/_T) v^2 = 8^2 + 8.4^2 - 2*8*8.4*cos(72°)Calculate v^2: Calculate the value of v^2 using the given values. v^2 = 64 + 70.56 - 2*8*8.4*cos(72°) To find cos(72°), we can use a calculator. cos(72°) ≈ 0.30901699 v^2 = 64 + 70.56 - 2*8*8.4*0.30901699 v^2 ≈ 134.56 - 2*8*8.4*0.30901699 v^2 ≈ 134.56 - 41.472 v^2 ≈ 93.088Find v length: Take the square root of v^2 to find the length of side v. v = √93.088 v ≈ 9.645 Now we have the lengths of all three sides of the triangle: t = 8 cm, u = 8.4 cm, and v ≈ 9.645 cm.Use Law of Sines: Use the Law of Sines to find the possible values of /_U. The Law of Sines states that for any triangle with sides a, b, and c, and angles A, B, and C opposite those sides, respectively, the following equation holds: (sin(A)/a) = (sin(B)/b) = (sin(C)/c). We can set up the equation to solve for /_U as follows: sin(/_T)/t = sin(/_U)/u sin(72°)/8 = sin(/_U)/8.4Calculate sin(/_U): Calculate sin(/_U) using the values we have. sin(/_U) = (sin(72°)/8) * 8.4 sin(72°) ≈ 0.95105652 sin(/_U) ≈ (0.95105652/8) * 8.4 sin(/_U) ≈ 0.95105652 * 1.05 sin(/_U) ≈ 0.99870934Find possible /_U values: Find the possible values of /_U by taking the arcsin of sin(/_U). /_U ≈ arcsin(0.99870934) Since the sine function has a range of [-1, 1] and is positive in the first and second quadrants, there are two possible angles for /_U: one in the first quadrant and one in the second quadrant. However, since we are dealing with a triangle, the angle must be less than 180° and greater than 0°. Therefore, we only consider the angle in the first quadrant. /_U ≈ arcsin(0.99870934) /_U ≈ 86.6° (to the nearest tenth of a degree)

In $\triangle \mathrm{TUV}, u=8.4 \mathrm{~cm}, t=8 \mathrm{~cm}$ and $\angle \mathrm{T}=72^{\circ}$ . Find all possible values of $\angle \mathrm{U}$ , to the nearest $10$ th of a degree. $\newline$ Answer:

Full solution

More problems from Find trigonometric functions using a calculator

Related topics

In △TUV,u=8.4 cm,t=8 cm \triangle \mathrm{TUV}, u=8.4 \mathrm{~cm}, t=8 \mathrm{~cm} △TUV,u=8.4 cm,t=8 cm and ∠T=72∘ \angle \mathrm{T}=72^{\circ} ∠T=72∘. Find all possible values of ∠U \angle \mathrm{U} ∠U, to the nearest 101010th of a degree.\newlineAnswer:

In $\triangle \mathrm{TUV}, u=8.4 \mathrm{~cm}, t=8 \mathrm{~cm}$ and $\angle \mathrm{T}=72^{\circ}$ . Find all possible values of $\angle \mathrm{U}$ , to the nearest $10$ th of a degree. $\newline$ Answer: