Welcome to Bytelearn!

Let’s check out your problem:

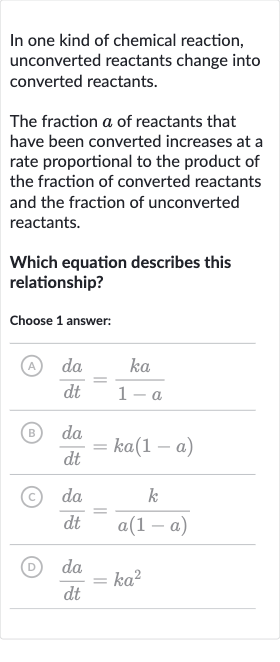
In one kind of chemical reaction, unconverted reactants change into converted reactants.$\newline$The fraction $a$ of reactants that have been converted increases at a rate proportional to the product of the fraction of converted reactants and the fraction of unconverted reactants.$\newline$Which equation describes this relationship?$\newline$Choose $1$ answer:$\newline$(A) $\frac{d a}{d t}=\frac{k a}{1-a}$$\newline$(B) $\frac{d a}{d t}=k a(1-a)$$\newline$(c) $\frac{d a}{d t}=\frac{k}{a(1-a)}$$\newline$(D) $\frac{d a}{d t}=k a^{2}$