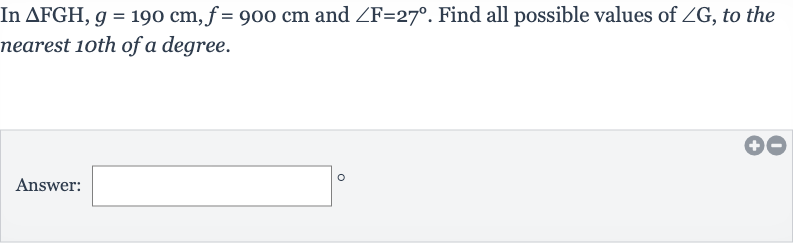
In /_\FGH,g=190cm,f=900cm and /_F=27^(@). Find all possible values of /_G, to the nearest 1oth of a degree. Answer:

Question

In  /_\FGH,g=190cm,f=900cm and  /_F=27^(@). Find all possible values of  /_G, to the nearest 1oth of a degree. Answer:

Bytelearn · Accepted Answer

Law of Sines Formula: To find the possible values of angle G, we can use the Law of Sines, which states that the ratio of the length of a side of a triangle to the sine of the opposite angle is constant for all sides and angles in the triangle. The formula is: $$ \frac{a}{\sin(A)} = \frac{b}{\sin(B)} = \frac{c}{\sin(C)} $$ where $ a, b, c $ are the lengths of the sides, and $ A, B, C $ are the opposite angles.Calculate Sine of Angle F: First, we need to find the sine of angle F, which is given as 27 degrees. We can use a calculator to find this value. $$ \sin(27^\circ) \approx 0.4540 $$Set Up Ratio with Law of Sines: Now, we can set up the ratio using the Law of Sines with the given sides g and f, and the known angle F. $$ \frac{f}{\sin(F)} = \frac{g}{\sin(G)} $$ $$ \frac{900}{\sin(27^\circ)} = \frac{190}{\sin(G)} $$Solve for Sine of Angle G: Next, we solve for $ \sin(G) $. $$ \sin(G) = \frac{190 \cdot \sin(27^\circ)}{900} $$ $$ \sin(G) \approx \frac{190 \cdot 0.4540}{900} $$ $$ \sin(G) \approx \frac{86.26}{900} $$ $$ \sin(G) \approx 0.09584 $$Find Angle G using Inverse Sine: To find angle G, we take the inverse sine (arcsin) of $ \sin(G) $. $$ G \approx \arcsin(0.09584) $$ Using a calculator, we find that: $$ G \approx 5.5^\circ $$Check Second Possible Value for Angle G: However, since the sine function is positive in both the first and second quadrants, there could be another possible value for angle G in the second quadrant. To find this, we use the fact that $ \sin(180^\circ - G) = \sin(G) $. $$ 180^\circ - G \approx 180^\circ - 5.5^\circ $$ $$ 180^\circ - G \approx 174.5^\circ $$Validate Valid Solution for Angle G: We must check if this second possible value for angle G is valid in the context of a triangle. The sum of angles in any triangle is 180 degrees. Since we already have angle F as 27 degrees, the sum of angles G and the third angle H must be $ 180^\circ - 27^\circ = 153^\circ $. If angle G were 174.5 degrees, the sum of angles G and H would exceed 180 degrees, which is not possible in a triangle. Therefore, the only valid solution for angle G is the one in the first quadrant.

In $\triangle \mathrm{FGH}, g=190 \mathrm{~cm}, f=900 \mathrm{~cm}$ and $\angle \mathrm{F}=27^{\circ}$ . Find all possible values of $\angle \mathrm{G}$ , to the nearest $10$ th of a degree. $\newline$ Answer:

Full solution

More problems from Find trigonometric functions using a calculator

Related topics

In △FGH,g=190 cm,f=900 cm \triangle \mathrm{FGH}, g=190 \mathrm{~cm}, f=900 \mathrm{~cm} △FGH,g=190 cm,f=900 cm and ∠F=27∘ \angle \mathrm{F}=27^{\circ} ∠F=27∘. Find all possible values of ∠G \angle \mathrm{G} ∠G, to the nearest 101010th of a degree.\newlineAnswer:

In $\triangle \mathrm{FGH}, g=190 \mathrm{~cm}, f=900 \mathrm{~cm}$ and $\angle \mathrm{F}=27^{\circ}$ . Find all possible values of $\angle \mathrm{G}$ , to the nearest $10$ th of a degree. $\newline$ Answer: