Full solution
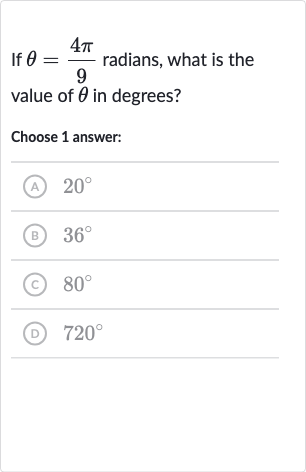
Q. If radians, what is the value of in degrees?Choose answer:(A) (B) (C) (D)
- Conversion Factor: To convert radians to degrees, we use the conversion factor that radians is equal to degrees. The question prompt is: "What is the value of in degrees when radians?"
- Multiply by Conversion Factor: We multiply by the conversion factor to convert from radians to degrees: .
- Cancel out : The in the numerator and the in the denominator cancel out, leaving us with .
- Calculate Multiplication: We calculate the multiplication: .
- Final Result: Divide by to get , and then multiply by : .