AI tutor
Full solution
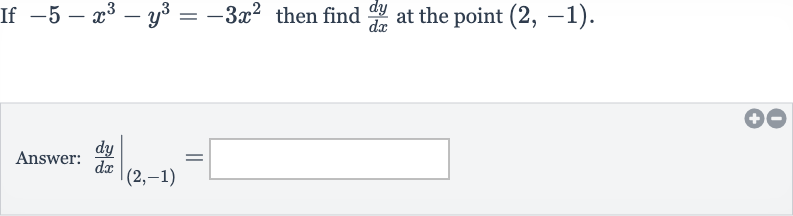
Q. If then find at the point .Answer:
- Differentiate Equation: First, we need to find the derivative of both sides of the equation with respect to to find . The equation is . Differentiate both sides with respect to , remembering to use the chain rule for the term since is a function of . This gives us:
- Solve for : Now, we need to solve for .
y^\left(\frac{dy}{dx}\right) = x + x^
Divide both sides by y^ to isolate .
= \frac{x + x^}{y^} - Substitute Point: Next, we substitute the point into the equation to find the value of at that point.This simplifies to:
- Calculate Value: Finally, we calculate the value.