Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

If -3x-7y=-1 is a true equation, what would be the value of -3(-3x-7y) ? Answer:

If −3x−7y=−1 -\mathbf{3 x}-\mathbf{7 y}=-\mathbf{1} is a true equation, what would be the value of −3(−3x−7y) -3(-3 x-7 y) ?\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Find trigonometric ratios using multiple identitiesright chevron icon

Full solution

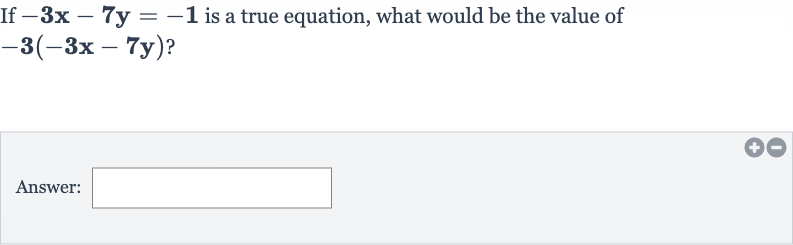
Q. If −3x−7y=−1 -\mathbf{3 x}-\mathbf{7 y}=-\mathbf{1} is a true equation, what would be the value of −3(−3x−7y) -3(-3 x-7 y) ?\newlineAnswer:
  1. Apply Distributive Property: We are given the equation −3x−7y=−1-3x - 7y = -1. We need to find the value of −3(−3x−7y)-3(-3x - 7y).\newlineFirst, we apply the distributive property to −3(−3x−7y)-3(-3x - 7y).\newline−3(−3x)−3(−7y)=3x+21y-3(-3x) - 3(-7y) = 3x + 21y.
  2. Substitute Given Equation: Now, we substitute the given equation −3x−7y=−1-3x - 7y = -1 into the expression 3x+21y3x + 21y. Since −3x−7y-3x - 7y equals −1-1, multiplying the entire equation by −3-3 will give us the value of 3x+21y3x + 21y. −3(−3x−7y)=−3(−1)-3(-3x - 7y) = -3(-1).
  3. Perform Multiplication: We perform the multiplication −3-3 times −1-1.−3(−1)=3-3(-1) = 3.
  4. Final Value: We have found the value of −3(−3x−7y)-3(-3x - 7y), which is 33.

More problems from Find trigonometric ratios using multiple identities

Question
If cos⁡(θ)=817 \cos(\theta)=\frac{8}{17} and 0∘<θ<90∘ 0^\circ<\theta<90^\circ , what is sec⁡(θ) \sec(\theta) ? Write your answer in simplified, rationalized form. sec⁡(θ)= \sec(\theta)= ______
Get tutor helpright-arrow

Posted 5 months ago

Question
cos⁡(x)=−0.3\cos(x) = -0.3. What is sin⁡(90∘−x)\sin(90^\circ - x)? ____
Get tutor helpright-arrow

Posted 9 months ago

Question
If csc⁡(θ)=178 \csc(\theta) = \frac{17}{8} and 0∘<θ<90∘ 0^\circ < \theta < 90^\circ , what is tan⁡(θ) \tan(\theta) ?\newlineWrite your answer in simplified, rationalized form.\newlinetan⁡(θ)= \tan(\theta) = ______\newline
Get tutor helpright-arrow

Posted 9 months ago

Question
Is the sine function even or odd?\newlineChoices:\newline[A]even\text{[A]even}\newline[B]odd\text{[B]odd}
Get tutor helpright-arrow

Posted 9 months ago

Question
Write the sentence as an equation. \newline8989 is equal to the quotient of yy and 1717 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 7 months ago

Question
Write the sentence as an equation. \newline4545 is equal to the quotient of zz and 55 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for hh. \newline h2+24h=0h^2 + 24h = 0 \newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas. \newline h=h = _____
Get tutor helpright-arrow

Posted 10 months ago

Question
h2−4h=0h^2 - 4h = 0
Get tutor helpright-arrow

Posted 7 months ago

Question
Combine the like terms to create an equivalent expression:\newline−k−(−8k)=□-k-(-8k)=\square
Get tutor helpright-arrow

Posted 10 months ago

Question
Which of the following degree measures is equal to 5π 5 \pi radians?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) 144∘ 144^{\circ} \newline(B) 900∘ 900^{\circ} \newline(C) 1,080∘ 1,080^{\circ} \newline(D) 1,800∘ 1,800^{\circ}
Get tutor helpright-arrow

Posted 7 months ago

View all (68)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant