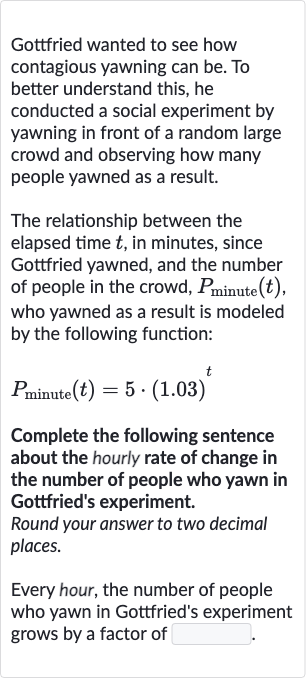
Gottfried wanted to see how contagious yawning can be. To better understand this, he conducted a social experiment by yawning in front of a random large crowd and observing how many people yawned as a result. The relationship between the elapsed time t, in minutes, since Gottfried yawned, and the number of people in the crowd, P_("minute ")(t), who yawned as a result is modeled by the following function: P_("minute ")(t)=5*(1.03)^(t) Complete the following sentence about the hourly rate of change in the number of people who yawn in Gottfried's experiment. Round your answer to two decimal places. Every hour, the number of people who yawn in Gottfried's experiment grows by a factor of

Question

Gottfried wanted to see how contagious yawning can be. To better understand this, he conducted a social experiment by yawning in front of a random large crowd and observing how many people yawned as a result. The relationship between the elapsed time  t, in minutes, since Gottfried yawned, and the number of people in the crowd,  P_("minute ")(t), who yawned as a result is modeled by the following function:  P_("minute ")(t)=5*(1.03)^(t) Complete the following sentence about the hourly rate of change in the number of people who yawn in Gottfried's experiment. Round your answer to two decimal places. Every hour, the number of people who yawn in Gottfried's experiment grows by a factor of

Bytelearn · Accepted Answer

Understand function and task: Understand the function and what is being asked. We are given the function P_(＂minute ＂)(t)=5*(1.03)^(t), which models the number of people yawning over time in minutes. We need to find the hourly rate of change, which means we need to determine how much the number of people yawning increases every hour.Convert time to hours: Convert the time from minutes to hours. Since there are 60 minutes in an hour, we need to find the factor by which the number of people yawning increases after 60 minutes.Substitute and calculate: Substitute t = 60 into the function to find the hourly rate of change. P_(＂minute ＂)(60) = 5*(1.03)^(60) Now we need to calculate (1.03)^(60).Calculate (1.03)^(60): Calculate (1.03)^(60) using a calculator. (1.03)^(60) ≈ 5.892Multiply to find total: Multiply the result by the initial number of people (5) to find the number of people who yawn after one hour. P_(＂minute ＂)(60) = 5 * 5.892 P_(＂minute ＂)(60) ≈ 29.46Determine hourly rate: Determine the hourly rate of change. The hourly rate of change is the factor by which the number of people increases every hour. Since we started with 5 people and ended up with approximately 29.46 people after one hour, we divide the final amount by the initial amount to find the rate of change. Hourly rate of change = P_(＂minute ＂)(60) / 5 Hourly rate of change ≈ 29.46 / 5 Hourly rate of change ≈ 5.892Round to two decimal: Round the hourly rate of change to two decimal places. Hourly rate of change ≈ 5.89 (rounded to two decimal places)

Full solution

More problems from Solve a system of equations using any method: word problems

Related topics