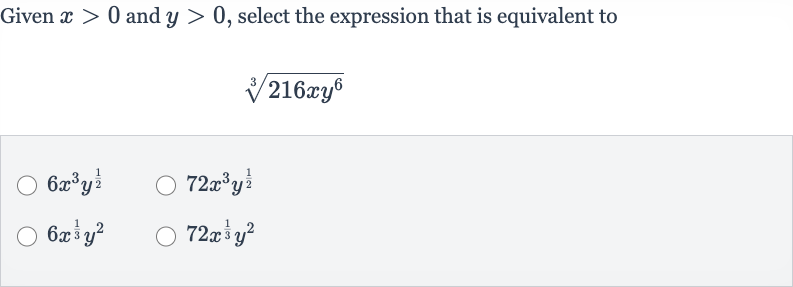Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Given
x > 0 and
y > 0, select the expression that is equivalent to

root(3)(216 xy^(6))

6x^(3)y^((1)/(2))

72x^(3)y^((1)/(2))

6x^((1)/(3))y^(2)

72x^((1)/(3))y^(2)

Given $x>0$ and $y>0$ , select the expression that is equivalent to $\newline$ $\sqrt[3]{216 x y^{6}}$ $\newline$ $6 x^{3} y^{\frac{1}{2}}$ $\newline$ $72 x^{3} y^{\frac{1}{2}}$ $\newline$ $6 x^{\frac{1}{3}} y^{2}$ $\newline$ $72 x^{\frac{1}{3}} y^{2}$

View step-by-step help

View step-by-step help

Multiplication with rational exponents

Full solution

Q. Given

x>0

and

y>0

, select the expression that is equivalent to

\newline

\sqrt[3]{216 x y^{6}}

\newline

6 x^{3} y^{\frac{1}{2}}

\newline

72 x^{3} y^{\frac{1}{2}}

\newline

6 x^{\frac{1}{3}} y^{2}

\newline

72 x^{\frac{1}{3}} y^{2}

Find Cube Root of $216$ : Simplify the cube root of $216$ . We know that $216$ is a perfect cube, as $216 = 6^3$ . Therefore, the cube root of $216$ is $6$ .
Separate Product of Variables: Simplify the cube root of $xy^6$ . Since $x > 0$ and $y > 0$ , we can separate the cube root of the product into the product of the cube roots: $\sqrt[3]{xy^6} = \sqrt[3]{x} \cdot \sqrt[3]{y^6}$ .
Simplify $y^6$ : Simplify the cube root of $y^6$ . We know that $y^6$ can be written as $(y^2)^3$ , which means the cube root of $y^6$ is $y^2$ .
Combine Results: Combine the simplified cube roots. $\newline$ Now we combine the results from Step $1$ and Step $3$ with the cube root of $x$ from Step $2$ : $6 \cdot \sqrt[3]{x} \cdot y^2$ .
Write Final Expression: Write the final expression. $\newline$ The final expression is $6x^{(1/3)}y^2$ .

More problems from Multiplication with rational exponents

Question

\newline

81^{\frac{1}{2}}

\newline

Posted 3 months ago

Question

\newline

(-32)^{1/5}

\newline

Posted 3 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 3 months ago

Question

Simplify. Assume all variables are positive.

\newline

(2r^{\frac{1}{3}})^8

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 2 months ago

Question

Simplify. Assume all variables are positive.

\newline

v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 3 months ago

Question

\newline

1^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}

\newline

Posted 2 months ago

Question

\newline

32^{(-1/5)}

Posted 2 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 3 months ago

Question

Simplify. Assume all variables are positive.

\newline

(27x)^{\frac{2}{3}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 2 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 2 months ago