Full solution
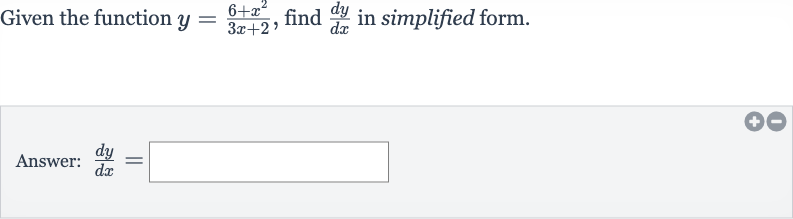
Q. Given the function , find in simplified form.Answer:
- Identify Function: Identify the function to differentiate.We are given the function . We need to find the derivative of this function with respect to , which is denoted as .
- Apply Quotient Rule: Apply the quotient rule for differentiation.The quotient rule states that the derivative of a function in the form of is given by . Here, and .
- Differentiate and : Differentiate and with respect to . The derivative of with respect to is . The derivative of with respect to is .
- Apply Derivatives to Quotient Rule: Apply the derivatives found in Step to the quotient rule.
- Simplify Expression: Simplify the expression.
- Check for Factorization: Check for any possible simplification or factorization. The numerator and the denominator do not have any common factors, so the expression is already in its simplest form.