Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

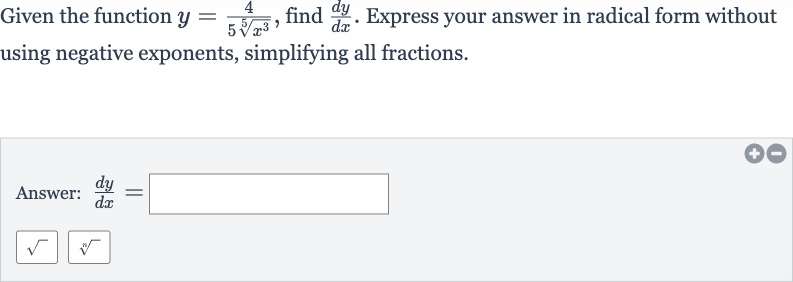
$Given the function y=(4)/(5root(5)(x^(3))), find (dy)/(dx). Express your answer in radical form without using negative exponents, simplifying all fractions. Answer: (dy)/(dx)=$

Given the function $y=\frac{4}{5 \sqrt[5]{x^{3}}}$ , find $\frac{d y}{d x}$ . Express your answer in radical form without using negative exponents, simplifying all fractions. $\newline$ Answer: $\frac{d y}{d x}=$

View step-by-step help

Home

Math Problems

Precalculus

Find trigonometric ratios using multiple identities

Full solution

Q. Given the function

y=\frac{4}{5 \sqrt[5]{x^{3}}}

, find

\frac{d y}{d x}

. Express your answer in radical form without using negative exponents, simplifying all fractions.

\newline

Answer:

\frac{d y}{d x}=

Rewrite function with fractional exponent: We are given the function $y = \frac{4}{5\sqrt{5}(x^{3})}$ . To find the derivative $\frac{dy}{dx}$ , we will use the power rule for differentiation, which states that if $y = x^n$ , then $\frac{dy}{dx} = n\cdot x^{n-1}$ . In this case, we need to rewrite the function in a form that allows us to apply the power rule.
Combine constants and powers: First, we rewrite the square root in the denominator as a fractional exponent: $\sqrt{5} = 5^{1/2}$ . Then, we rewrite the function $y$ as $y = \frac{4}{5 \cdot 5^{1/2} \cdot x^3}$ . This can be further simplified by combining the constants and the powers of $5$ .
Apply power rule for differentiation: The function becomes $y = (\frac{4}{5^{\frac{3}{2}}}) \cdot x^{-3}$ . Now we can apply the power rule to find the derivative with respect to $x$ .
Simplify the derivative expression: Differentiating $y$ with respect to $x$ , we get $\frac{dy}{dx} = \frac{4}{5^{\frac{3}{2}}} \cdot (-3) \cdot x^{(-3 - 1)}$ . This simplifies to $\frac{dy}{dx} = -\frac{12}{5^{\frac{3}{2}}} \cdot x^{-4}$ .
Rewrite in radical form: We want to express the answer in radical form without using negative exponents. To do this, we rewrite $x^{-4}$ as $1/x^4$ and $5^{3/2}$ as $\sqrt{5^3}$ or $\sqrt{125}$ .
Finalize the derivative expression: The derivative $\frac{dy}{dx}$ in radical form without negative exponents is $\frac{dy}{dx} = -\frac{12}{\sqrt{125}*x^4}$ . We can simplify $\sqrt{125}$ to $5\sqrt{5}$ .
Finalize the derivative expression: The derivative $(dy)/(dx)$ in radical form without negative exponents is $(dy)/(dx) = -12/(\sqrt{125}*x^4)$ . We can simplify $\sqrt{125}$ to $5\sqrt{5}$ .Finally, we simplify the fraction by dividing $-12$ by $5\sqrt{5}$ . The derivative $(dy)/(dx)$ is $(dy)/(dx) = -12/(5\sqrt{5}*x^4)$ .