Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

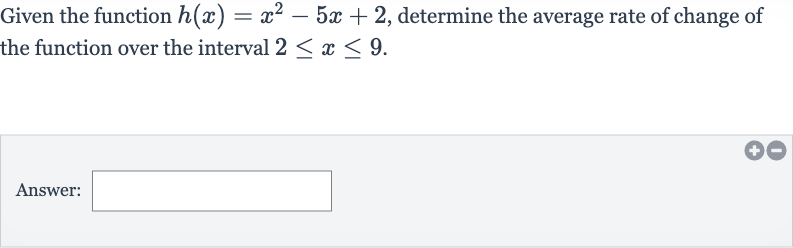
Given the function h(x)=x^(2)-5x+2, determine the average rate of change of the function over the interval 2 <= x <= 9. Answer:

Given the function h(x)=x2−5x+2 h(x)=x^{2}-5 x+2 , determine the average rate of change of the function over the interval 2≤x≤9 2 \leq x \leq 9 .\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Average rate of changeright chevron icon

Full solution

Q. Given the function h(x)=x2−5x+2 h(x)=x^{2}-5 x+2 , determine the average rate of change of the function over the interval 2≤x≤9 2 \leq x \leq 9 .\newlineAnswer:
  1. Calculate Lower Endpoint: To find the average rate of change of the function h(x)=x2−5x+2h(x) = x^2 - 5x + 2 over the interval [2,9][2, 9], we need to calculate the difference in the function values at the endpoints of the interval and divide by the difference in the xx-values.
  2. Calculate Upper Endpoint: First, we calculate the value of h(x)h(x) at the lower endpoint of the interval, which is x=2x = 2.\newlineh(2)=(2)2−5(2)+2=4−10+2=−4h(2) = (2)^2 - 5(2) + 2 = 4 - 10 + 2 = -4.
  3. Find Average Rate of Change: Next, we calculate the value of h(x)h(x) at the upper endpoint of the interval, which is x=9x = 9. h(9)=(9)2−5(9)+2=81−45+2=38h(9) = (9)^2 - 5(9) + 2 = 81 - 45 + 2 = 38.
  4. Find Average Rate of Change: Next, we calculate the value of h(x)h(x) at the upper endpoint of the interval, which is x=9x = 9. h(9)=(9)2−5(9)+2=81−45+2=38h(9) = (9)^2 - 5(9) + 2 = 81 - 45 + 2 = 38. Now, we find the average rate of change by subtracting the function value at the lower endpoint from the function value at the upper endpoint and dividing by the difference in xx-values. Average rate of change = (h(9)−h(2))/(9−2)=(38−(−4))/(9−2)=42/7=6(h(9) - h(2)) / (9 - 2) = (38 - (-4)) / (9 - 2) = 42 / 7 = 6.

More problems from Average rate of change

Question
What is the domain of this function?\newline(9,–2)(6,–10)(–3,9)(5,2)(9,–2)(6,–10)(–3,9)(5,2)\newlineChoices:\newline(A){–3,5,6,9}(B){–10,–2,2,9}(C){2,5,6,9}(D){–6,–3,5,9}(A)\{–3,5,6,9\}\newline(B)\{–10,–2,2,9\}\newline(C)\{2,5,6,9\}\newline(D)\{–6,–3,5,9\}
Get tutor helpright-arrow

Posted 1 month ago

Question
Look at this set of ordered pairs:\newline(2,14)(2, 14)\newline(10,12)(10, 12)\newline(3,−19)(3, -19)\newlineIs this relation a function?\newlineChoices:\newline[[yes]\text{[[yes]}[no]]\text{[no]]}
Get tutor helpright-arrow

Posted 1 month ago

Question
Use the following function rule to find f(11) f(11) .\newlinef(x)=−10−7x f(x) = -10 - 7x \newlinef(11)= f(11) = _____
Get tutor helpright-arrow

Posted 1 month ago

Question
Find the slope of the line y=−3x−25 y = -3x - \frac{2}{5} .\newlineSimplify your answer and write it as a proper fraction, improper fraction, or integer.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 1 month ago

Question
A line has a slope of 33 and passes through the point (−2,−10)(-2,-10). Write its equation in slope-intercept form.\newlineWrite your answer using integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 1 month ago

Question
What is the range of this function?\newliney = |x|\newlineChoices:\newlineall real numbers\text{all real numbers}\newline{y∣y≥0}\{y \mid y \geq 0\}\newline{y∣y≤0}\{y \mid y \leq 0\}\newline{y∣y>0}\{y \mid y > 0\}
Get tutor helpright-arrow

Posted 2 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 1 1 unit down of f(x)=∣x∣ f(x) = |x| .\newlineWrite your answer in the form a∣x−h∣+k a|x - h| + k , where a a , h h , and k k are integers.\newlineg(x)= g(x) = ______\newline
Get tutor helpright-arrow

Posted 2 months ago

Question
What is the domain of this function?\newline(8,–1)(7,–11)(–4,10)(4,3)(8,–1)(7,–11)(–4,10)(4,3)\newlineChoices: \newline(A){–4,4,7,8}(A)\{–4,4,7,8\}\newline(B){–11,–1,3,10}(B)\{–11,–1,3,10\}\newline(C){3,4,7,8}(C)\{3,4,7,8\}\newline(D){–7,–4,4,8}(D)\{–7,–4,4,8\}
Get tutor helpright-arrow

Posted 3 months ago

Question
What is the domain of this function?\newline(10, –3)(2, –9)(–5, 8)(3, 1)(10,\,–3)(2,\,–9)(–5,\,8)(3,\,1)\newlineChoices:\newline[{–5,2,3,10}][{–9, –3,1,8}][{1,2,3,10}][{–10, –5,2,3}][\{–5,2,3,10\}][\{–9,\,–3,1,8\}][\{1,2,3,10\}][\{–10,\,–5,2,3\}]
Get tutor helpright-arrow

Posted 1 month ago

Question
What is the domain of this function?\newline(1,–4)(9,–12)(–6,11)(7,5)(1,–4)(9,–12)(–6,11)(7,5)\newlineChoices:(A){–6,1,7,9}\newline\\(A)\{–6,1,7,9\}\newline(B){–12,–4,5,11}(B)\{–12,–4,5,11\}\newline(C{5,1,7,9}(C\{5,1,7,9\}\newline(D){–9,–6,1,7}(D)\{–9,–6,1,7\}
Get tutor helpright-arrow

Posted 1 month ago

View all (25)