AI tutor
Full solution
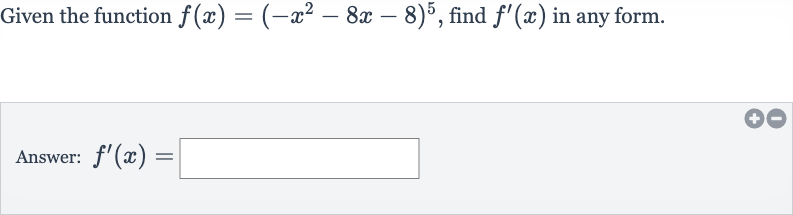
Q. Given the function , find in any form.Answer:
- Identify Functions: Identify the outer function and the inner function for the application of the chain rule.The outer function is and the inner function is .
- Apply Chain Rule: Apply the chain rule which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.Let , then .The chain rule gives us .
- Calculate Derivative: Calculate the derivative of the inner function with respect to .
- Substitute into Formula: Substitute the derivative of the inner function and the inner function itself into the chain rule formula. .
- Simplify Expression: Simplify the expression if necessary.In this case, the expression is already in a simplified form, so we can state the final answer..
More problems from Find the vertex of the transformed function
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help