Full solution
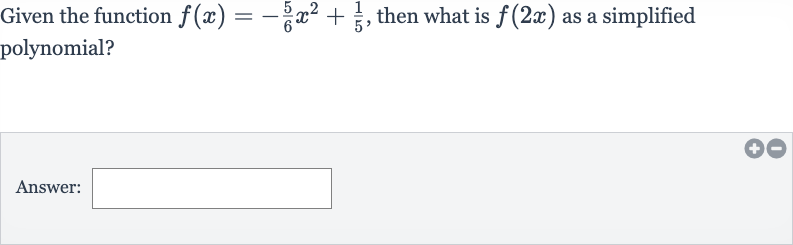
Q. Given the function , then what is as a simplified polynomial?Answer:
- Substitute in : Substitute into the function . We need to replace every instance of in the function with .
- Simplify square of : Simplify the square of . We need to square the term to get .
- Substitute squared term: Substitute the squared term back into the function.Now we replace with in the function.
- Multiply coefficients: Multiply the coefficients.We need to multiply the coefficient by .
- Write simplified polynomial: Write the simplified polynomial.Now we have the coefficient for , we can write the simplified polynomial.
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help