Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

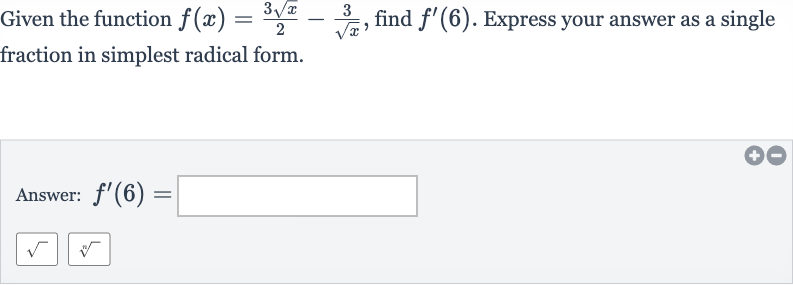
$Given the function f(x)=(3sqrtx)/(2)-(3)/(sqrtx), find f^(')(6). Express your answer as a single fraction in simplest radical form. Answer: f^(')(6)=$

Given the function $f(x)=\frac{3 \sqrt{x}}{2}-\frac{3}{\sqrt{x}}$ , find $f^{\prime}(6)$ . Express your answer as a single fraction in simplest radical form. $\newline$ Answer: $f^{\prime}(6)=$

View step-by-step help

Home

Math Problems

Precalculus

Find trigonometric ratios using multiple identities

Full solution

Q. Given the function

f(x)=\frac{3 \sqrt{x}}{2}-\frac{3}{\sqrt{x}}

, find

f^{\prime}(6)

. Express your answer as a single fraction in simplest radical form.

\newline

Answer:

f^{\prime}(6)=

Find Derivative of Function: First, we need to find the derivative of the function $f(x) = \frac{3\sqrt{x}}{2} - \frac{3}{\sqrt{x}}$ . To do this, we will use the power rule for differentiation. The square root of $x$ can be written as $x^{1/2}$ , so the function becomes $f(x) = \frac{3}{2}x^{1/2} - 3x^{-1/2}$ .
Differentiate First Term: Now, let's differentiate the first term $(\frac{3}{2})x^{(\frac{1}{2})}$ . Using the power rule, the derivative of $x^{n}$ is $n\cdot x^{(n-1)}$ , so the derivative of $(\frac{3}{2})x^{(\frac{1}{2})}$ is $(\frac{3}{2})\cdot(\frac{1}{2})\cdot x^{(\frac{1}{2} - 1)} = (\frac{3}{4})x^{(-\frac{1}{2})}$ .
Differentiate Second Term: Next, we differentiate the second term $-3x^{-1/2}$ . Again, using the power rule, the derivative of $-3x^{-1/2}$ is $-3*(-1/2)*x^{-1/2 - 1} = (3/2)x^{-3/2}$ .
Combine Derivatives: Combining the derivatives of both terms, we get the derivative of the function $f(x)$ , which is $f'(x) = \frac{3}{4}x^{-\frac{1}{2}} + \frac{3}{2}x^{-\frac{3}{2}}$ .
Evaluate at $x=6$ : Now we need to evaluate the derivative at $x=6$ . Substituting $x$ with $6$ , we get $f'(6) = (\frac{3}{4})(6)^{-\frac{1}{2}} + (\frac{3}{2})(6)^{-\frac{3}{2}}$ .
Simplify Square Root and Cube Root: To simplify, we calculate the square root and the cube root of $6$ . The square root of $6$ is $\sqrt{6}$ , and the cube root of $6$ is $6^{3/2} = (\sqrt{6})^3$ . So, $f'(6) = \frac{3}{4}(\frac{1}{\sqrt{6}}) + \frac{3}{2}(\frac{1}{(\sqrt{6})^3})$ .
Find Common Denominator: We can simplify the expression further by finding a common denominator. The common denominator for $\sqrt{6}$ and $(\sqrt{6})^3$ is $(\sqrt{6})^3$ . So, we multiply the first term by $\frac{\sqrt{6}}{\sqrt{6}}$ to get the common denominator. f'(\(6) = \left(\frac{ $3$ }{ $4$ }\right)\left(\frac{\sqrt{ $6$ }}{\sqrt{ $6$ }}\right)\left(\frac{ $1$ }{\sqrt{ $6$ }}\right) + \left(\frac{ $3$ }{ $2$ }\right)\left(\frac{ $1$ }{(\sqrt{ $6$ })^ $3$ }\right) = \frac{ $3$ \sqrt{ $6$ }}{ $4$ \sqrt{ $6$ }^ $2$ } + \left(\frac{ $3$ }{ $2$ }\right)\left(\frac{ $1$ }{\sqrt{ $6$ }^ $3$ }\right).
Combine Terms with Common Denominator: Simplifying the denominators, we have $\sqrt{6^2} = 6$ and $\sqrt{6^3} = 6\sqrt{6}$ . So, $f'(6) = \frac{3\sqrt{6}}{4\cdot 6} + \frac{3}{2}\left(\frac{1}{6\sqrt{6}}\right) = \frac{3\sqrt{6}}{24} + \frac{3}{2}\left(\frac{1}{6\sqrt{6}}\right).$
Simplify Fraction: Now, we combine the terms over a common denominator, which is $24\sqrt{6}$ . To do this, we multiply the second term by $\frac{4}{4}$ to get the common denominator. $f'(6) = \frac{3\sqrt{6}}{24} + \frac{3}{2}\left(\frac{4}{24\sqrt{6}}\right) = \frac{3\sqrt{6}}{24} + \frac{6}{24\sqrt{6}}$ .
Factor Out $3$ from Numerator: Adding the two fractions together, we get $f'(6) = \frac{3\sqrt{6} + 6}{24\sqrt{6}}$ . This is the derivative of the function $f(x)$ evaluated at $x=6$ .
Factor Out $3$ from Numerator: Adding the two fractions together, we get $f'(6) = \frac{3\sqrt{6} + 6}{24\sqrt{6}}$ . This is the derivative of the function $f(x)$ evaluated at $x=6$ .Finally, we simplify the fraction by factoring out a $3$ from the numerator. $f'(6) = \frac{3(\sqrt{6} + 2)}{24\sqrt{6}}$ . We can simplify this further by dividing both the numerator and the denominator by $3$ . $f'(6) = \frac{\sqrt{6} + 2}{8\sqrt{6}}$ .