$g(x)=(x+12)(x-4)$ $\newline$ The function $g$ is defined by the given equation. What is the minimum value of $g(x) ?$

View step-by-step help

Home

Math Problems

Algebra 2

Find the vertex of the transformed function

Full solution

g(x)=(x+12)(x-4)

\newline

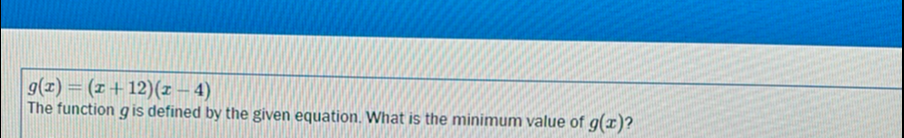
The function

g

is defined by the given equation. What is the minimum value of

g(x) ?

Expand and Express in Standard Form: We have the function $g(x) = (x + 12)(x - 4)$ . To find the minimum value of $g(x)$ , we need to find the vertex of the parabola represented by this function. Since the coefficient of $x^2$ is positive, the parabola opens upwards, and the vertex will give us the minimum value.
Complete the Square: First, we need to express $g(x)$ in standard form, which is $y = ax^2 + bx + c$ . We do this by expanding the given equation. $\newline$ $g(x) = (x + 12)(x - 4) = x^2 - 4x + 12x - 48 = x^2 + 8x - 48$
Rewrite in Vertex Form: Next, we complete the square to rewrite the function in vertex form, which is $y = a(x - h)^2 + k$ , where $(h, k)$ is the vertex of the parabola. $\newline$ To complete the square, we take the coefficient of $x$ , which is $8$ , divide it by $2$ , and square it. $(\frac{8}{2})^2 = 4^2 = 16$ . $\newline$ We then add and subtract this number inside the equation to complete the square. $\newline$ $g(x) = x^2 + 8x + 16 - 16 - 48$
Identify Vertex: Now, we can rewrite the equation with the completed square and simplify the constants. $\newline$ g(x) = $(x^2 + 8x + 16) - 64$ $\newline$ g(x) = $(x + 4)^2 - 64$
Find Minimum Value: The vertex form of the equation is now $g(x) = (x + 4)^2 - 64$ . The vertex $(h, k)$ of this parabola is $(-4, -64)$ . Since the parabola opens upwards ( $a$ is positive), the vertex represents the minimum point of the function.
Find Minimum Value: The vertex form of the equation is now $g(x) = (x + 4)^2 - 64$ . The vertex $(h, k)$ of this parabola is $(-4, -64)$ . Since the parabola opens upwards ( $a$ is positive), the vertex represents the minimum point of the function. The minimum value of $g(x)$ is the y-coordinate of the vertex, which is $-64$ .