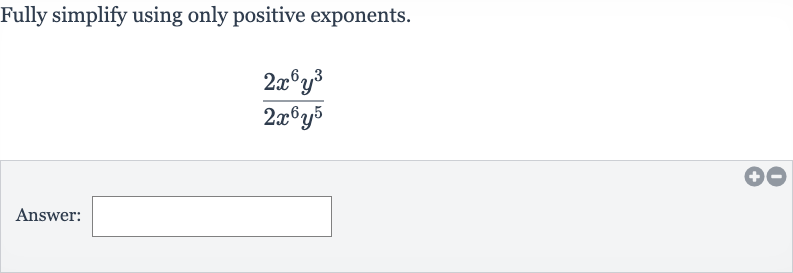Full solution
Q. Fully simplify using only positive exponents.Answer:
- Identify Like Terms: Write down the expression and identify like terms in the numerator and the denominator.We have like terms in the numerator and the denominator: , , and raised to some power.
- Cancel Common Terms: Cancel out the common terms in the numerator and the denominator.The in the numerator and denominator cancel each other out, as do the terms.
- Apply Quotient Rule: Apply the quotient rule for exponents to simplify the expression.The quotient rule states that when dividing like bases, you subtract the exponents.
- Rewrite with Positive Exponents: Since we need to express the answer with only positive exponents, we rewrite as .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help