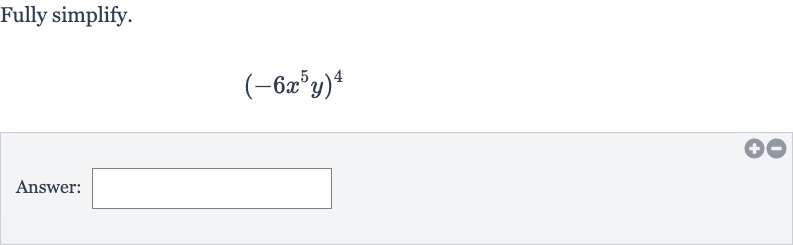AI tutor
Full solution
Q. Fully simplify.Answer:
- Identify base and exponent: Identify the base and the exponent in .In , the base is and the exponent is .
- Apply power of product rule: Apply the power of a product rule, which states that , to the base and exponent.
- Calculate : Calculate each part separately.First, calculate ., since the negative sign will become positive when raised to an even power.
- Calculate : Now, calculate ., using the power of a power rule which states that .
- Calculate : Finally, calculate .
- Combine calculated parts: Combine all the calculated parts to get the final simplified expression.
More problems from Evaluate rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help