AI tutor
Full solution
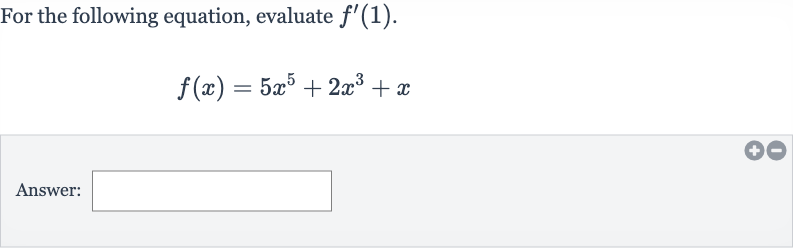
Q. For the following equation, evaluate .Answer:
- Identify Function: We need to find the derivative of the function with respect to . This will give us , which we can then evaluate at .
- Apply Power Rule: Differentiate the function term by term using the power rule, which states that the derivative of with respect to is .
- Evaluate at : Evaluate the derivative at .
More problems from Find the vertex of the transformed function
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help