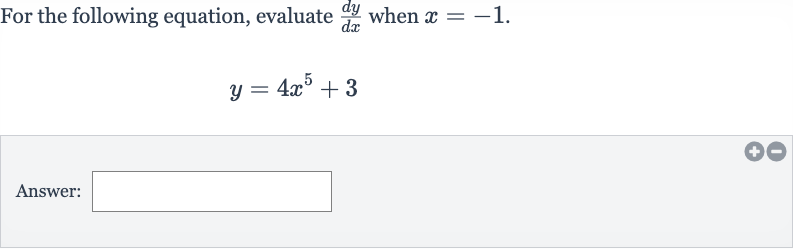Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

For the following equation, evaluate (dy)/(dx) when x=-1. y=4x^(5)+3 Answer:

For the following equation, evaluate dydx \frac{d y}{d x} when x=−1 x=-1 .\newliney=4x5+3 y=4 x^{5}+3 \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Evaluate a function: plug in an expressionright chevron icon

Full solution

Q. For the following equation, evaluate dydx \frac{d y}{d x} when x=−1 x=-1 .\newliney=4x5+3 y=4 x^{5}+3 \newlineAnswer:
  1. Identify Equation and Derivative: Identify the equation and the derivative we need to find.\newlineWe are given the equation y=4x5+3y = 4x^{5} + 3 and we need to find the derivative of yy with respect to xx, which is denoted as dydx\frac{dy}{dx}.
  2. Differentiate with Respect to x: Differentiate the equation with respect to x.\newlineTo find (dy)/(dx)(dy)/(dx), we need to differentiate each term of the equation y=4x5+3y = 4x^{5} + 3 with respect to x.\newlineThe derivative of 4x54x^{5} with respect to x is 20x420x^{4} (using the power rule: d/dx[xn]=nx(n−1)d/dx [x^n] = nx^{(n-1)}).\newlineThe derivative of a constant, like 33, is 00.\newlineSo, (dy)/(dx)=20x4+0(dy)/(dx) = 20x^{4} + 0, which simplifies to (dy)/(dx)=20x4(dy)/(dx) = 20x^{4}.
  3. Evaluate at x=−1x = -1: Evaluate the derivative at x=−1x = -1.\newlineNow that we have the derivative, we can substitute x=−1x = -1 into dydx=20x4\frac{dy}{dx} = 20x^{4} to find the value of the derivative at that point.\newlinedydx\frac{dy}{dx} at x=−1x = -1 is 20(−1)420(-1)^{4}.\newlineSince (−1)4(-1)^{4} is 11, this simplifies to dydx\frac{dy}{dx} at x=−1x = -1 is x=−1x = -111, which is x=−1x = -122.

More problems from Evaluate a function: plug in an expression

Question
Look at this set of ordered pairs: (3,18)(15,9)(13,14)(18,12)(3, 18) (15, 9) (13, 14) (18, 12) Is this relation a function?? Choices:[[yes][[\text{yes}][no]][\text{no}]]
Get tutor helpright-arrow

Posted 1 month ago

Question
Use the following function rule to find g(b+2) g(b + 2) . \newlineSimplify your answer. \newlineg(a)=4a+8 g(a) = 4a + 8 g(b+2)= g(b + 2) = ______
Get tutor helpright-arrow

Posted 2 months ago

Question
Thanks to an online typing course, Audrey has become a much faster typist. The function f(x) f(x) gives how many words she can type in x x seconds.\newlineWhat does f(45)=68 f(45) = 68 tell you?\newlineChoices:\newline(A) Audrey can type 68 words in 45 seconds.\text{Audrey can type \( 68 \) words in \( 45 \) seconds.} \newline(B) Audrey can type 45 words in 68 seconds.\text{Audrey can type \( 45 \) words in \( 68 \) seconds.}
Get tutor helpright-arrow

Posted 1 month ago

Question
What is the domain of this relation? \newline(4,−3)(−3,−10)(−5,5)(9,−2)(9,−1)(4,-3) (-3,-10) (-5,5) (9,-2) (9,-1) \newlineChoices: \newline(A) {−5,−3,4,9}\{-5,-3,4,9\} \newline(B) {−5,−3,4,5,9}\{-5,-3,4,5,9\} \newline(C) {−3,−2,4,9}\{-3,-2,4,9\} \newline(D) {−3,−2,5,9}\{-3,-2,5,9\}
Get tutor helpright-arrow

Posted 1 month ago

Question
Every day at lunch, Vicky swims laps in the pool for one hour. The faster her speed, the more laps she is able to swim in the hour. s=s = the speed at which Vicky swims l=l = the number of laps Vicky is able to swim in one hour Which of the variables is independent and which is dependent?\newlineChoices:\newline[A]s is the independent variable and l is the dependent variable [A] s \text{ is the independent variable and } l \text{ is the dependent variable } \newline[B]l is the independent variable and s is the dependent variable [B] l \text{ is the independent variable and } s \text{ is the dependent variable }
Get tutor helpright-arrow

Posted 2 months ago

Question
Use the following function rule to find f(4) f(4) .\newlinef(x)=9−x f(x) = 9 - x \newlinef(4)= f(4) =
Get tutor helpright-arrow

Posted 1 month ago

Question
Simplify to create an equivalent expression.\newline−2(−5n+6)+5(2−7n)-2(-5n+6)+5(2-7n)\newlineChoose 11 answer:\newline(A) −2-2\newline(B) −25n+10-25n+10\newline(C) −25n+2-25n+2\newline(D) −25n−2-25n-2
Get tutor helpright-arrow

Posted 4 months ago

Question
Simplify to create an equivalent expression.\newline3n+2(−2n−1)3n+2(-2n-1)\newlineChoose 11 answer:\newline(A) −n+2-n+2\newline(B) n+2n+2\newline(C) −n−2-n-2\newline(D) n−2n-2
Get tutor helpright-arrow

Posted 4 months ago

Question
Simplify to create an equivalent expression.\newline5(10k+1)+2(2+8k)5(10k+1)+2(2+8k)\newlineChoose 11 answer:\newline(A) 66k+966k+9\newline(B) 64k+964k+9\newline(C) 66k+5466k+54\newline(D) 64k−964k-9
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify to create an equivalent expression.\newline5+7(8r−2)5+7(8r-2)\newlineChoose 11 answer:\newline(A) 56r+356r+3\newline(B) 56r+956r+9\newline(C) 8r−98r-9\newline(D) 56r−956r-9
Get tutor helpright-arrow

Posted 4 months ago

View all (17)