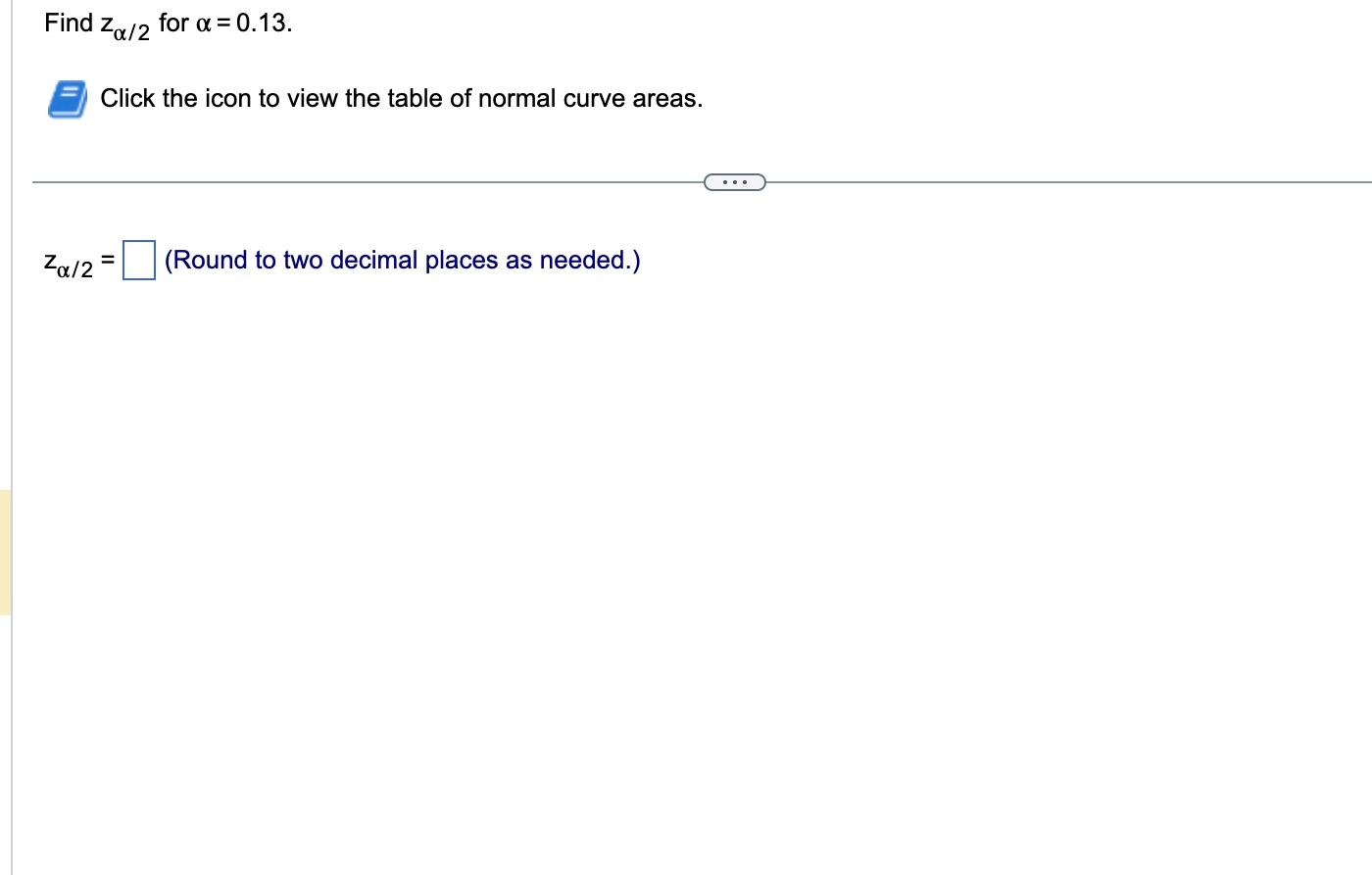
Find z_(alpha//2) for alpha=0.13. z_(alpha//2)=◻ (Round to two decimal places as needed.)

Question

Bytelearn · Accepted Answer

Understand the problem: Understand the problem. We need to find the z-score (z_(alpha//2)) that corresponds to an alpha level of 0.13 for a two-tailed test. This means we are looking for the z-score where the area in each tail of the standard normal distribution is 0.13/2, since the total area for both tails must add up to alpha.Calculate alpha/2: Calculate alpha/2. To find the z-score for the two-tailed test, we first need to divide alpha by 2 because the alpha level is split between the two tails of the distribution. alpha/2 = 0.13 / 2 = 0.065Use z-table: Use the standard normal distribution table. We will use the standard normal distribution table (z-table) to find the z-score that corresponds to an area of 0.065 to the left of the z-score. Since the table typically gives the area to the left of the z-score, we need to find the value that corresponds to 1 - 0.065 = 0.935, because we want the area in the right tail to be 0.065.Find z-score: Find the z-score from the table. Looking at the z-table, we find the closest area to 0.935 and then identify the corresponding z-score. The z-score that corresponds to an area of 0.935 is approximately 1.51.Round z-score: Round the z-score to two decimal places. The z-score we found is already at two decimal places, so no further rounding is necessary. z_(alpha//2) = 1.51

Find $z_{\alpha / 2}$ for $\alpha=0.13$ . $\newline$ $\mathrm{z}_{\alpha / 2}=\square$ (Round to two decimal places as needed.)

Full solution

More problems from Find trigonometric functions using a calculator

Related topics

Find zα/2 z_{\alpha / 2} zα/2​ for α=0.13 \alpha=0.13 α=0.13.\newlinezα/2=□ \mathrm{z}_{\alpha / 2}=\square zα/2​=□ (Round to two decimal places as needed.)

Find $z_{\alpha / 2}$ for $\alpha=0.13$ . $\newline$ $\mathrm{z}_{\alpha / 2}=\square$ (Round to two decimal places as needed.)