Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the zeros of the function f(x)=2x^(2)-8.4 x+5.2. Round values to the nearest hundredth (if necessary). Answer: x=

Find the zeros of the function f(x)=2x2−8.4x+5.2 f(x)=2 x^{2}-8.4 x+5.2 . Round values to the nearest hundredth (if necessary).\newlineAnswer: x= x=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Find trigonometric functions using a calculatorright chevron icon

Full solution

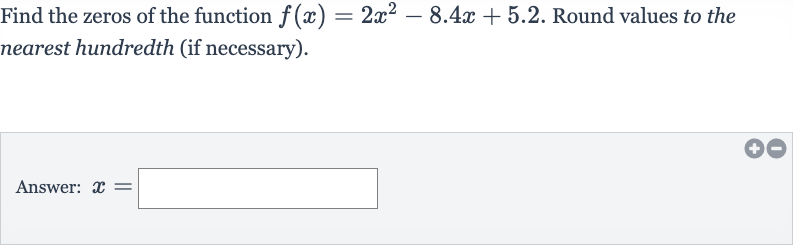
Q. Find the zeros of the function f(x)=2x2−8.4x+5.2 f(x)=2 x^{2}-8.4 x+5.2 . Round values to the nearest hundredth (if necessary).\newlineAnswer: x= x=
  1. Identify Equation Type: Identify the type of equation and the method to find its zeros.\newlineThe given function f(x)=2x2−8.4x+5.2f(x) = 2x^2 - 8.4x + 5.2 is a quadratic equation. To find the zeros of a quadratic equation, we can use the quadratic formula: x=−b±b2−4ac2ax = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}, where aa, bb, and cc are the coefficients of the equation ax2+bx+c=0ax^2 + bx + c = 0.
  2. Apply Quadratic Formula: Apply the quadratic formula to the given equation.\newlineFor the equation f(x)=2x2−8.4x+5.2f(x) = 2x^2 - 8.4x + 5.2, the coefficients are a=2a = 2, b=−8.4b = -8.4, and c=5.2c = 5.2. Plugging these values into the quadratic formula gives us:\newlinex=−(−8.4)±(−8.4)2−4⋅2⋅5.22⋅2x = \frac{-(-8.4) \pm \sqrt{(-8.4)^2 - 4 \cdot 2 \cdot 5.2}}{2 \cdot 2}\newlinex=8.4±70.56−41.64x = \frac{8.4 \pm \sqrt{70.56 - 41.6}}{4}\newlinex=8.4±28.964x = \frac{8.4 \pm \sqrt{28.96}}{4}
  3. Simplify Expression and Calculate: Simplify the expression under the square root and calculate the zeros.\newlinex=8.4±28.964x = \frac{8.4 \pm \sqrt{28.96}}{4}\newlinex=8.4±5.384x = \frac{8.4 \pm 5.38}{4}\newlineNow we have two possible solutions for xx:\newlinex1=8.4+5.384x_1 = \frac{8.4 + 5.38}{4}\newlinex2=8.4−5.384x_2 = \frac{8.4 - 5.38}{4}
  4. Calculate First Zero: Calculate the first zero x1x_1.x1=8.4+5.384x_1 = \frac{8.4 + 5.38}{4}x1=13.784x_1 = \frac{13.78}{4}x1=3.445x_1 = 3.445Rounded to the nearest hundredth, x1≈3.45x_1 \approx 3.45
  5. Calculate Second Zero: Calculate the second zero (x2x_2).\newlinex2=(8.4−5.38)/4x_2 = (8.4 - 5.38) / 4\newlinex2=3.02/4x_2 = 3.02 / 4\newlinex2=0.755x_2 = 0.755\newlineRounded to the nearest hundredth, x2≈0.76x_2 \approx 0.76

More problems from Find trigonometric functions using a calculator

Question
Find all solutions with 0∘≤θ≤180∘0^\circ \leq \theta \leq 180^\circ. Give the exact answer(s) in simplest form. If there are multiple answers, separate them with commas.\newlinecos⁡(θ)=−1\cos(\theta) = -1\newline‾∘\underline{\hspace{2em}}^\circ
Get tutor helpright-arrow

Posted 7 months ago

Question
Kimi's school is 1515 kilometers west of her house and 88 kilometers south of her friend Franklin's house. Every day, Kimi bicycles from her house to her school. After school, she bicycles from her school to Franklin's house. Before dinner, she bicycles home on a bike path that goes straight from Franklin's house to her own house. How far does Kimi bicycle each day?\newline_____\_\_\_\_\_ kilometers
Get tutor helpright-arrow

Posted 7 months ago

Question
Evaluate. Write your answer as an integer or as a decimal rounded to the nearest hundredth.\newlinecos⁡35∘=\cos 35^\circ = __
Get tutor helpright-arrow

Posted 7 months ago

Question
Find all solutions with −90∘≤θ≤90∘-90^\circ \leq \theta \leq 90^\circ. Give the exact answer(s) in simplest form. If there are multiple answers, separate them with commas.\newlinecsc⁡(θ)=−1\csc(\theta) = -1\newline____ ∘\_\_\_\_\,^\circ
Get tutor helpright-arrow

Posted 8 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecot⁡30∘=\cot 30^\circ = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
The terminal side of an angle θ\theta in standard position intersects the unit circle at (8485,1385)(\frac{84}{85}, \frac{13}{85}). What is cos⁡(θ)\cos(\theta)?\newlineWrite your answer in simplified, rationalized form.\newline______
Get tutor helpright-arrow

Posted 7 months ago

Question
−90∘<θ<90∘-90^\circ < \theta < 90^\circ. Find the value of θ\theta in degrees.\newlinetan⁡(θ)=0\tan(\theta) = 0\newlineWrite your answer in simplified, rationalized form. Do not round.\newlineθ=\theta = ____∘^\circ\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecsc⁡60∘=\csc 60^\circ = ______
Get tutor helpright-arrow

Posted 8 months ago

Question
θ\theta is an acute angle. Find the value of θ\theta in degrees.\newlinesec⁡(θ)=2\sec(\theta) = \sqrt{2}\newlineθ=\theta = ____°\degree
Get tutor helpright-arrow

Posted 7 months ago

Question
Evaluate. Write your answer in simplified, rationalized form. Do not round.\newlinecos⁡90∘=\cos 90^\circ = ____
Get tutor helpright-arrow

Posted 9 months ago

View all (217)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant