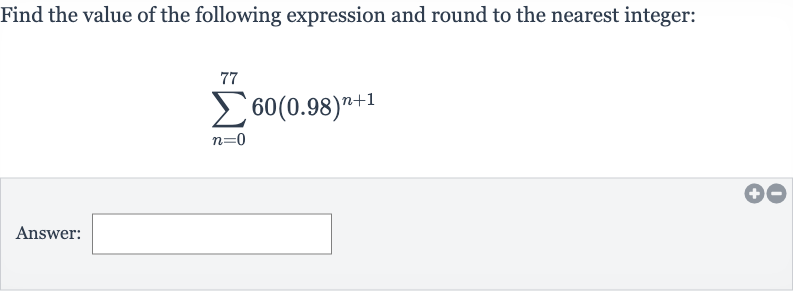
Find the value of the following expression and round to the nearest integer: sum_(n=0)^(77)60(0.98)^(n+1) Answer:

Question

Find the value of the following expression and round to the nearest integer:  sum_(n=0)^(77)60(0.98)^(n+1) Answer:

Bytelearn · Accepted Answer

Given geometric series: We are given a geometric series with the first term a = 60 * 0.98 and the common ratio r = 0.98. The sum of a finite geometric series can be found using the formula S = a(1 - r^n) / (1 - r), where n is the number of terms. In this case, we have 78 terms (from n=0 to n=77).Calculate first term: First, calculate the first term of the series: a = 60 * 0.98^(1) = 60 * 0.98.Calculate common ratio: Now, calculate the common ratio r = 0.98.Calculate number of terms: Next, calculate the number of terms n = 77 - 0 + 1 = 78.Apply sum formula: Now, apply the formula for the sum of a finite geometric series: S = a(1 - r^n) / (1 - r). Substitute a = 60 * 0.98, r = 0.98, and n = 78 into the formula.Perform calculation: Perform the calculation: S = (60 * 0.98)(1 - 0.98^78) / (1 - 0.98).Calculate 0.98^78: Calculate 0.98^78 using a calculator to avoid any potential math errors due to the small size of the number.Find numerator: Subtract 0.98^78 from 1 to find the numerator of the sum formula.Calculate denominator: Calculate the denominator of the sum formula, which is 1 - 0.98.Find sum: Divide the numerator by the denominator to find the sum S.Round to nearest integer: Round the sum S to the nearest integer to get the final answer.

Find the value of the following expression and round to the nearest integer: $\newline$ $\sum_{n=0}^{77} 60(0.98)^{n+1}$ $\newline$ Answer:

Full solution

More problems from Sum of finite series starts from 1

Related topics

Find the value of the following expression and round to the nearest integer:\newline∑n=07760(0.98)n+1 \sum_{n=0}^{77} 60(0.98)^{n+1} n=0∑77​60(0.98)n+1\newlineAnswer:

Find the value of the following expression and round to the nearest integer: $\newline$ $\sum_{n=0}^{77} 60(0.98)^{n+1}$ $\newline$ Answer: