Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the value of the following expression and round to the nearest integer: sum_(n=0)^(25)100(1.24)^(n+1) Answer:

Find the value of the following expression and round to the nearest integer:\newline∑n=025100(1.24)n+1 \sum_{n=0}^{25} 100(1.24)^{n+1} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Sum of finite series starts from 1right chevron icon

Full solution

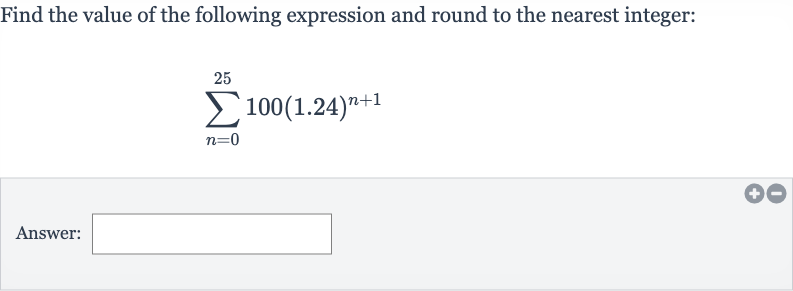
Q. Find the value of the following expression and round to the nearest integer:\newline∑n=025100(1.24)n+1 \sum_{n=0}^{25} 100(1.24)^{n+1} \newlineAnswer:
  1. Given Geometric Series: We are given a geometric series with the first term a=100×1.24a = 100 \times 1.24 and the common ratio r=1.24r = 1.24. The sum of the first kk terms of a geometric series is given by the formula Sk=a×(1−rk)/(1−r)S_k = a \times (1 - r^k) / (1 - r) when rr is not equal to 11. Here, we need to find the sum of the series from n=0n = 0 to n=25n = 25, which means we will be summing 2626 terms.
  2. Calculate First Term: First, calculate the first term of the series a=100×1.24a = 100 \times 1.24.
  3. Apply Sum Formula: The first term aa is 124124.
  4. Substitute Values: Now, apply the formula for the sum of the first kk terms of a geometric series: Sk=a⋅(1−rk)/(1−r)S_k = a \cdot (1 - r^k) / (1 - r). Here, k=26k = 26, a=124a = 124, and r=1.24r = 1.24.
  5. Calculate Exponent: Substitute the values into the formula to get S26=124×(1−1.2426)/(1−1.24)S_{26} = 124 \times (1 - 1.24^{26}) / (1 - 1.24).
  6. Substitute Exponent: Calculate 1.24261.24^{26} using a calculator to avoid any potential math errors.
  7. Simplify Expression: The value of 1.24261.24^{26} is approximately 99.53299.532. This is a large number, so it's important to use a calculator to ensure accuracy.
  8. Calculate Sum: Now, substitute 99.53299.532 back into the formula to get S26=124×(1−99.532)/(1−1.24)S_{26} = 124 \times (1 - 99.532) / (1 - 1.24).
  9. Round to Nearest Integer: Simplify the expression to find the sum S26=124×(−98.532)/(−0.24)S_{26} = 124 \times (-98.532) / (-0.24).
  10. Round to Nearest Integer: Simplify the expression to find the sum S26=124×(−98.532)/(−0.24)S_{26} = 124 \times (-98.532) / (-0.24).Calculate the sum S26=124×410.55S_{26} = 124 \times 410.55 (rounded to two decimal places for intermediate steps).
  11. Round to Nearest Integer: Simplify the expression to find the sum S26=124×(−98.532)/(−0.24)S_{26} = 124 \times (-98.532) / (-0.24).Calculate the sum S26=124×410.55S_{26} = 124 \times 410.55 (rounded to two decimal places for intermediate steps).The sum S26S_{26} is approximately 50908.250908.2.
  12. Round to Nearest Integer: Simplify the expression to find the sum S26=124×(−98.532)/(−0.24)S_{26} = 124 \times (-98.532) / (-0.24).Calculate the sum S26=124×410.55S_{26} = 124 \times 410.55 (rounded to two decimal places for intermediate steps).The sum S26S_{26} is approximately 50908.250908.2.Round 50908.250908.2 to the nearest integer to get the final answer.
  13. Round to Nearest Integer: Simplify the expression to find the sum S26=124×(−98.532)/(−0.24)S_{26} = 124 \times (-98.532) / (-0.24).Calculate the sum S26=124×410.55S_{26} = 124 \times 410.55 (rounded to two decimal places for intermediate steps).The sum S26S_{26} is approximately 50908.250908.2.Round 50908.250908.2 to the nearest integer to get the final answer.The rounded value is 5090850908.

More problems from Sum of finite series starts from 1

Question
Find the sum of the finite arithmetic series. ∑n=110(7n+4)\sum_{n=1}^{10} (7n+4)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`
Get tutor helpright-arrow

Posted 5 months ago

Question
Type the missing number in this sequence: `2, 4 8`, ______,`32`
Get tutor helpright-arrow

Posted 5 months ago

Question
What kind of sequence is this? 2,10,50,250,…2, 10, 50, 250, \ldots Choices:Choices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
What is the missing number in this pattern? 1,4,9,16,25,36,49,64,81,____1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_
Get tutor helpright-arrow

Posted 7 months ago

Question
Classify the series. ∑n=012(n+2)3 \sum_{n = 0}^{12} (n + 2)^3 \newlineChoices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the first three partial sums of the series.\newline1+6+11+16+21+26+⋯1 + 6 + 11 + 16 + 21 + 26 + \cdots\newlineWrite your answers as integers or fractions in simplest form.\newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the third partial sum of the series. \newline3+9+15+21+27+33+⋯3 + 9 + 15 + 21 + 27 + 33 + \cdots\newlineWrite your answer as an integer or a fraction in simplest form. \newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the first three partial sums of the series. \newline1+7+13+19+25+31+⋯1 + 7 + 13 + 19 + 25 + 31 + \cdots\newlineWrite your answers as integers or fractions in simplest form. \newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 9 months ago

Question
Does the infinite geometric series converge or diverge?\newline1+34+916+2764+⋯1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots\newlineChoices:\newline[A]converge\text{[A]converge}\newline[B]diverge\text{[B]diverge}
Get tutor helpright-arrow

Posted 5 months ago

View all (132)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant