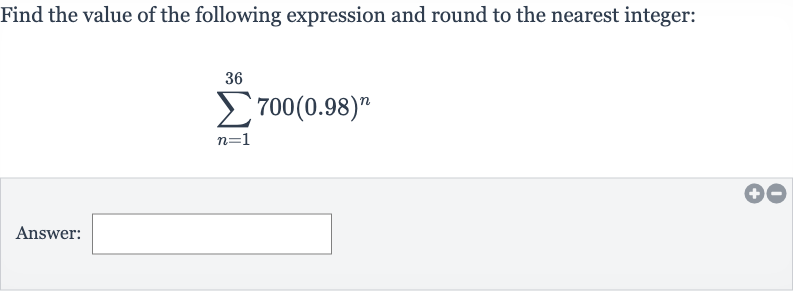
Find the value of the following expression and round to the nearest integer: sum_(n=1)^(36)700(0.98)^(n) Answer:

Question

Find the value of the following expression and round to the nearest integer:  sum_(n=1)^(36)700(0.98)^(n) Answer:

Bytelearn · Accepted Answer

Recognize Geometric Series: Recognize that the given expression is a geometric series. The general form of a geometric series is sum_(n=0)^(infinity) ar^n, where a is the first term, r is the common ratio, and n is the term number. In this case, a = 700 and r = 0.98.Use Sum Formula: Use the formula for the sum of the first n terms of a geometric series, which is S_n = a(1 - r^n) / (1 - r), where S_n is the sum of the first n terms. Here, n = 36, a = 700, and r = 0.98. We will plug these values into the formula to find the sum.Calculate with Values: Calculate the sum using the formula. S_36 = 700(1 - 0.98^36) / (1 - 0.98) First, calculate 0.98^36 using a calculator.Calculate 0.98^36: Calculate 0.98^36. 0.98^36 ≈ 0.4873 (rounded to four decimal places for simplicity)Substitute Back: Substitute 0.98^36 back into the sum formula. S_36 = 700(1 - 0.4873) / (1 - 0.98) Now, calculate 1 - 0.4873.Calculate 1 - 0.4873: Calculate 1 - 0.4873. 1 - 0.4873 = 0.5127Calculate Denominator: Substitute 0.5127 into the sum formula and calculate the denominator 1 - 0.98. S_36 = 700 * 0.5127 / (1 - 0.98) 1 - 0.98 = 0.02Calculate Sum: Calculate the sum S_36. S_36 = 700 * 0.5127 / 0.02 Now, perform the division and multiplication to find the sum.Perform Division: Perform the calculation. S_36 = 700 * 0.5127 / 0.02 S_36 = 700 * 25.635 S_36 = 17944.5Round to Nearest Integer: Round the sum to the nearest integer. The sum S_36 rounded to the nearest integer is 17945.

Find the value of the following expression and round to the nearest integer: $\newline$ $\sum_{n=1}^{36} 700(0.98)^{n}$ $\newline$ Answer:

Full solution

More problems from Sum of finite series starts from 1

Related topics

Find the value of the following expression and round to the nearest integer:\newline∑n=136700(0.98)n \sum_{n=1}^{36} 700(0.98)^{n} n=1∑36​700(0.98)n\newlineAnswer:

Find the value of the following expression and round to the nearest integer: $\newline$ $\sum_{n=1}^{36} 700(0.98)^{n}$ $\newline$ Answer: