Full solution
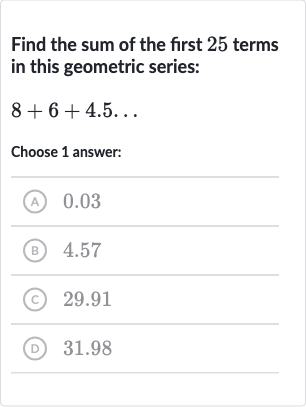
Q. Find the sum of the first terms in this geometric series:. Choose answer:(A) (B) (C) (D)
- Identify Terms and Values: First, identify the first term (), common ratio (), and number of terms () in the geometric series.The first term is , the second term is , and the third term is .To find the common ratio , we divide the second term by the first term: .The number of terms is given as .
- Calculate Common Ratio: Use the formula for the sum of the first terms of a geometric series: , where is the sum of the first terms.Plug in the values: .
- Use Sum Formula: Calculate the sum using the values from the previous step.