Full solution
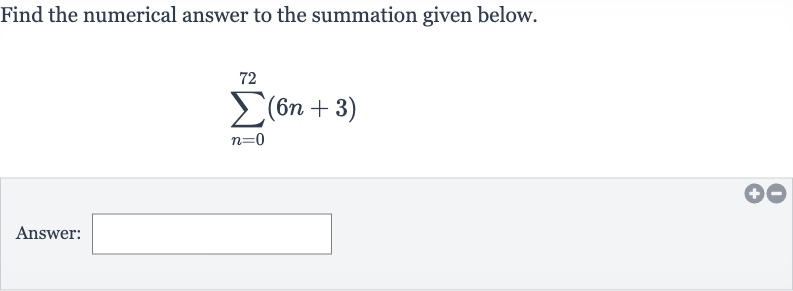
Q. Find the numerical answer to the summation given below.Answer:
- Calculate number of terms: We need to find the sum of the arithmetic series given by the formula from to . The general formula for the sum of an arithmetic series is , where is the number of terms, is the first term, and is the last term.
- Find first term: First, we calculate the number of terms in the series. Since we start at and go to , we have terms.
- Find last term: Next, we find the first term of the series by substituting into the formula . This gives us .
- Use sum formula: Now, we find the last term of the series by substituting into the formula . This gives us .
- Perform calculation: We can now use the sum formula for an arithmetic series: . Substituting the values we have, .
- Multiply by : Perform the calculation inside the parentheses first: .
- Perform final multiplication: Now, multiply by to find the sum of the series: .
- Perform final multiplication: Now, multiply by to find the sum of the series: .Finally, perform the multiplication to find the sum: .