Full solution
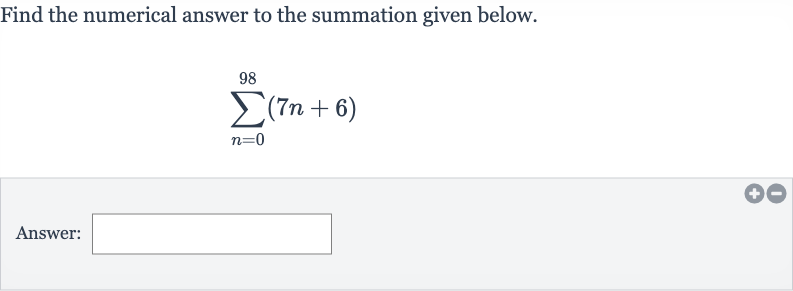
Q. Find the numerical answer to the summation given below.Answer:
- Recognize Linear Expression Split: Recognize that the summation is of a linear expression in terms of . The summation can be split into two separate summations: one for the term and one for the constant term .
- Apply Summation Rule Linear Term: Apply the summation rule for the first term, which is a linear term . The sum of an arithmetic series is given by the formula , where is the constant multiplier of . In this case, and .
- Calculate Sum First Term: Calculate the sum of the first term using the formula from Step .
- Apply Summation Rule Constant Term: Apply the summation rule for the second term, which is a constant term . The sum of a constant series is given by the formula , where is the constant term. In this case, and .
- Calculate Sum Second Term: Calculate the sum of the second term using the formula from Step .
- Add Final Results: Add the results from Step and Step to get the final answer.Final Sum = =