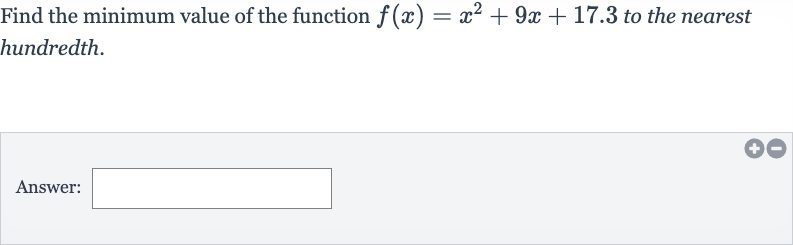
Find the minimum value of the function f(x)=x^(2)+9x+17.3 to the nearest hundredth. Answer:

Question

Find the minimum value of the function  f(x)=x^(2)+9x+17.3 to the nearest hundredth. Answer:

Bytelearn · Accepted Answer

Identify Coefficients: To find the minimum value of the quadratic function f(x) = x^2 + 9x + 17.3, we can complete the square or use the vertex formula for a parabola. The vertex form of a parabola is given by f(x) = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. Since the coefficient of x^2 is positive, the parabola opens upwards, and the vertex represents the minimum point.Calculate Vertex Coordinates: First, we identify the coefficients a, b, and c in the standard form of the quadratic equation, which is f(x) = ax^2 + bx + c. Here, a = 1, b = 9, and c = 17.3.Find x-coordinate of Vertex: The x-coordinate of the vertex (h) can be found using the formula h = -b/(2a). Plugging in the values of a and b, we get h = -9/(2*1) = -9/2 = -4.5.Find y-coordinate of Vertex: Now, we will find the y-coordinate of the vertex (k) by plugging the value of h back into the function. So, k = f(-4.5) = (-4.5)^2 + 9*(-4.5) + 17.3.Calculate Minimum Value: Calculating k, we get k = 20.25 - 40.5 + 17.3 = -20.25 + 17.3 = -2.95.Determine Vertex of Parabola: Therefore, the vertex of the parabola is at the point (-4.5, -2.95), and since this is a parabola that opens upwards, the y-coordinate of the vertex represents the minimum value of the function.Round to Nearest Hundredth: Rounding the minimum value to the nearest hundredth, we get -2.95 as the minimum value of the function f(x) = x^2 + 9x + 17.3.

Find the minimum value of the function $f(x)=x^{2}+9 x+17.3$ to the nearest hundredth. $\newline$ Answer:

Full solution

More problems from Find trigonometric functions using a calculator

Related topics

Find the minimum value of the function f(x)=x2+9x+17.3 f(x)=x^{2}+9 x+17.3 f(x)=x2+9x+17.3 to the nearest hundredth.\newlineAnswer:

Find the minimum value of the function $f(x)=x^{2}+9 x+17.3$ to the nearest hundredth. $\newline$ Answer: