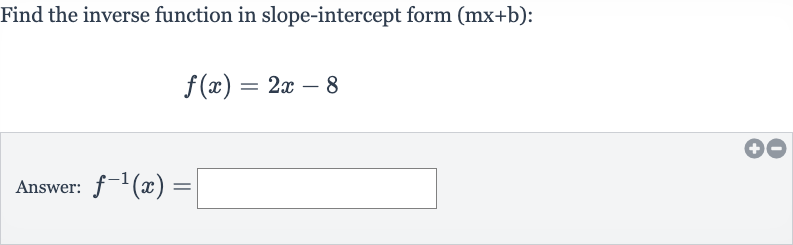
Find the inverse function in slope-intercept form $(\mathrm{mx}+\mathrm{b})$ : $\newline$ $f(x)=2 x-8$ $\newline$ Answer: $f^{-1}(x)=$

View step-by-step help

Home

Math Problems

Algebra 2

Find the vertex of the transformed function

Full solution

Q. Find the inverse function in slope-intercept form

(\mathrm{mx}+\mathrm{b})

\newline

f(x)=2 x-8

\newline

Answer:

f^{-1}(x)=

Understand Inverse Function Concept: Understand the concept of an inverse function. An inverse function essentially reverses the operation of the original function. If $f(x)$ takes an input $x$ and produces an output $y$ , then the inverse function $f^{-1}(x)$ takes $y$ as an input and produces the original $x$ as an output.
Write Original Function: Write the original function. $\newline$ The original function is $f(x) = 2x - 8$ . To find the inverse, we need to solve for $x$ in terms of $y$ .
Swap x and y: Swap x and y. $\newline$ To find the inverse function, we switch the roles of x and y. This means we will replace $f(x)$ with $y$ and then solve for $x$ . $\newline$ So, we have $y = 2x - 8$ .
Solve for x: Solve for x. $\newline$ Now we need to solve the equation $y = 2x - 8$ for $x$ . $\newline$ Add $8$ to both sides of the equation to isolate the term with $x$ on one side: $y + 8 = 2x$ .
Divide by $2$ : Divide by ext{ extdollar} $2$ ext{ extdollar}. $\newline$ To solve for ext{ extdollar}x ext{ extdollar}, divide both sides of the equation by ext{ extdollar} $2$ ext{ extdollar}: ext{ extdollar}rac{y + $8$ }{ $2$ } = x ext{ extdollar}.
Write Inverse Function: Write the inverse function. $\newline$ Now that we have $x$ in terms of $y$ , we can write the inverse function. Replace $x$ with $f^{-1}(x)$ and $y$ with $x$ to get the inverse function in terms of $x$ . $\newline$ $f^{-1}(x) = \frac{x + 8}{2}$ .
Convert to Slope-Intercept Form: Convert to slope-intercept form. $\newline$ The slope-intercept form is $y = mx + b$ . Our inverse function is already in this form, with $m = \frac{1}{2}$ and $b = \frac{8}{2}$ . $\newline$ So, $f^{-1}(x) = \frac{1}{2} \times x + 4$ .