Full solution
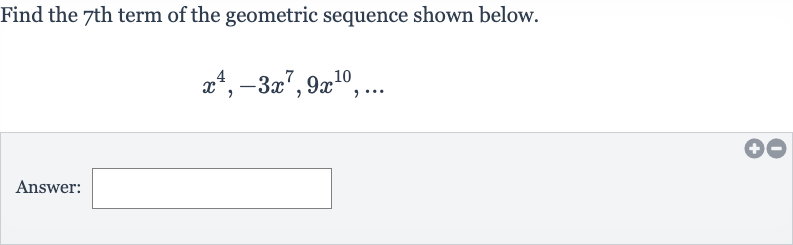
Q. Find the th term of the geometric sequence shown below.Answer:
- Find Common Ratio: To find the term of the geometric sequence, we first need to determine the common ratio () of the sequence. The common ratio can be found by dividing any term by the previous term.Let's divide the second term by the first term to find the common ratio.
- Calculate th Term: Now that we have the common ratio, we can find the th term using the formula for the nth term of a geometric sequence, which is , where is the first term and is the term number.The first term is , the common ratio is , and we want to find the th term .
- Simplify Expression: Now we need to simplify the expression for the th term.
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help