AI tutor
Full solution
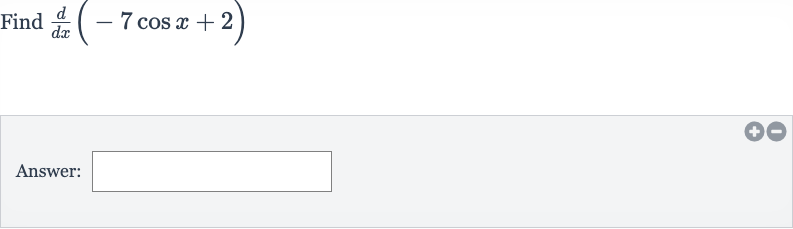
Q. Find Answer:
- Identify function: Identify the function to differentiate.We are given the function and we need to find its derivative with respect to .
- Apply rules: Apply the derivative rules.The derivative of a constant is , and the derivative of with respect to is . We will use these rules to differentiate each term of the function.
- Differentiate first term: Differentiate the first term.The first term is . The derivative of is , so the derivative of is times the derivative of , which is .
- Differentiate second term: Differentiate the second term.The second term is a constant, . The derivative of a constant is , so the derivative of with respect to is .
- Combine derivatives: Combine the derivatives of the terms.The derivative of the function is the sum of the derivatives of its terms, which is .
- Simplify result: Simplify the result.Since adding does not change the value, the final derivative of the function is simply .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help